Why Beauty is Truth (31 page)

More generally, the same line of argument proves that when
p
is an odd prime, the regular
p
-gon is constructible
if and only if p
– 1 is a power of 2. Such odd primes are called Fermat primes because Fermat was the first to investigate them. The Greeks knew of constructions for the regular 3-gon and the regular 5-gon. Observe that 3 –1 = 2, and 5 – 1 = 4, both powers of 2. So the Greek results are consistent with Gauss's criterion, and 3 and 5 are the first two Fermat primes. On the other hand, 7 – 1 = 6, not a power of two, so the regular 7-gon is not constructible.

A bit of extra work leads to Gauss's characterization: the regular
n
-gon is constructible if and only if
n
is a power of two, or a power of two multiplied by
distinct
Fermat primes.

This leaves the question, what are the Fermat primes? The next one after 3 and 5 is Gauss's discovery, 17. The next is 257, followed by the rather large number 65,537. These are the only known Fermat primes. It has never been proved that no further Fermat primes exist—but it has also never been proved that they don't. For all we know, there might be some absolutely gigantic Fermat prime not yet known to humanity. At
the current state of knowledge this number is at least 2
^33554432
+ 1, and indeed that might be the next Fermat prime. (The exponent 33554432 is itself a power of 2, namely 2
²⁵
. All Fermat primes are one greater than two raised to the power of
a power of
two.) This number has more than ten million digits. Even after Gauss's great discoveries, we still do not know for sure exactly which regular polygons are constructible, but the only gap in our knowledge is the possible existence of very large Fermat primes.

Although Gauss proved that the 17-gon is constructible, he did not actually describe the construction as such, though he did remark that the main point is to construct a line of length

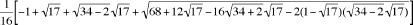
   The formula that determines Gauss's construction of the regular 17-gon.

Since square roots are always constructible, the required construction is implicit in this remarkable number. The first explicit construction was devised by Ulrich von Huguenin in 1803. H. W. Richmond found a simpler version in 1893.

In 1832, F. J. Richelot published a series of papers constructing the regular 257-gon, under the title “De resolutione algebraica aequationis x
²⁵⁷
= 1, sive de divisione circuli per bisectionem anguli septies repetitam in partes 257 inter se aequales commentatio coronata,” which is even more impressive than the number of sides of his polygon.

There is an apocryphal tale that an overzealous PhD student was assigned the construction of the 65537-gon as a thesis project, and reappeared with it twenty years later. The truth is almost as bizarre: J. Hermes, of the University of Lingen, devoted ten years to the task, finishing in 1894, and his unpublished work is preserved at the University of Göttingen. Unfortunately, John Horton Conway, perhaps the only mathematician to have looked at these documents in modern times, doubts that the work is correct.

W
illiam Rowan Hamilton was the greatest mathematician Ireland has ever produced. He was born at the stroke of midnight between 3 and 4 August 1805, and never quite made up his mind which of those dates was his birthday. Mostly he settled for the 3
^rd
, but his tombstone bears the date 4 August, because he switched to that date in later life for sentimental reasons. He was a brilliant linguist, a mathematical genius, and an alcoholic. He set out to invent an algebra of three dimensions but realized, in a flash of intuition that caused him to vandalize a bridge, that he would have to settle for four dimensions instead. He forever changed the human view of algebra, space, and time.

William was born into a wealthy family, the third son of Archibald Hamilton, a lawyer with a sound head for business. William also had a sister, Eliza. His father was partial to the odd glass or three, which made him good company for a while but a growing embarrassment as the evening wore on. Archibald was articulate, intelligent, and religious, and he passed on all of his significant traits, alcohol and all, to his youngest son. William's mother, Sarah Hutton, was even more intelligent than her husband, and came from a family of intellectual distinction, but her influence on young William, other than through her genes, was cut short when the father packed the boy off to be tutored by his uncle James at the age of three. James was a curate and an accomplished linguist, and his interests determined the main direction of William's education.

The results were impressive but obsessively narrow. By the age of five, William was fluent in Greek, Latin, and Hebrew. By eight he could speak French and Italian. Two years later he had added Arabic and Sanskrit; then Persian, Syrian, Hindu, Malay, Mahratti, and Bengali. Attempts to teach
the lad Chinese were stymied by a lack of suitable texts. James complained that “it cost me a large sum to supply him from London, but I hope the money was well expended.”

The mathematician and quasi-historian Eric Temple Bell (“quasi” because he never let an awkward fact get in the way of a good story) asked, “What was it all
for
?”

Fortunately for science and mathematics, William was saved from a life of mastering ever more of the world's thousands of languages when he came into contact with the American calculating prodigy Zerah Colburn. Colburn was one of those strange people who resemble a human pocket calculator; he had a talent for rapid, accurate computation. If you asked Colburn for the cube root of 1,860,867, he would reply “123” without pausing for breath.

This talent is distinct from mathematical ability, just as a facility for spelling does not make a good novelist. Except for Gauss, who left numerous big calculations in his notebooks and manuscripts, very few of the great mathematicians were lightning calculators. The rest were competent calculators—in those days you had to be—but no better than a qualified accountant. Even today, computers have not completely rendered pencil-and-paper calculations, or mental ones, obsolete; you can often gain insight into a mathematical problem by doing the calculations by hand and watching the symbols shuffle themselves around. But given the right software, much of it written by mathematicians, anyone with an hour's training can knock the socks off the likes of Colburn.

None of this will make you remotely resemble Gauss.

Colburn did not fully understand the tricks and short cuts he employed, though he was aware that memory played a big part. He was introduced to Hamilton in the expectation that the youthful genius would be able to shed light on these mysterious techniques. William did that and even came up with improvements. By the time Colburn departed, Hamilton had finally found a topic worthy of his astonishing brainpower.

By the age of seventeen, Hamilton had read many of the works of the mathematical masters, and knew enough mathematical astronomy to calculate eclipses. He still spent more time on the classics than on mathematics, but the latter had become his true passion. Soon he was making new discoveries. Just as the 19-year-old Gauss discovered the construction of a regular 17-gon, so the young Hamilton made an equally unprecedented breakthrough, an analogy—mathematically, an identity—between mechanics
and optics, the science of light. He first alluded to these ideas in a cryptic letter to his sister Eliza, but we can be fairly certain of their nature from a subsequent letter to his cousin Arthur.

The discovery was amazing. Mechanics is the study of moving bodies—cannonballs traveling in a parabolic arc, pendulums swinging regularly from side to side, and planets moving in ellipses round the Sun. Optics is about the geometry of light rays, reflection and refraction, rainbows and prisms and telescope lenses. That they were connected was a surprise; that they were
the same
was unbelievable.

It was also true. And it led directly to the formal setting used today by mathematicians and mathematical physicists, not just in mechanics and optics but in quantum theory too: Hamiltonian systems. Their main feature is that they derive the equations of motion of a mechanical system from a single quantity, the total energy, now called the
Hamiltonian
of the system. The resulting equations involve not just the positions of the parts of the system but how fast they are moving—the
momentum
of the system. Finally, the equations have the beautiful feature that they do not depend on the choice of coordinates. Beauty is truth, at least in mathematics. And here the physics is both beautiful and true.

Hamilton was luckier than either Abel or Galois in that his unusual talents were widely recognized from early childhood. So it was no surprise when, in 1823, he gained admission to Ireland's leading university, Trinity College Dublin. Nor was it a surprise to find him at the head of a field of one hundred candidates. At Trinity, he took all the prizes. More importantly, he finished the first volume of his masterpiece on optics.

In the spring of 1825, Hamilton discovered the attractions of the fairer sex, in the form of one Catherine Disney. Unwisely, he confined his attentions to writing poems, and his would-be love promptly married a wealthy clergyman, fifteen years her senior, who had a less literary approach to fair damsels. Hamilton was devastated; despite being staunchly religious, he thought about drowning himself, a mortal sin. Second thoughts prevailed, and he consoled himself by pouring out his frustrations in yet another poem.

Hamilton loved poetry, and his circle of friends included leading members of the literati. William Wordsworth became a close friend; he also spent time with Samuel Taylor Coleridge and various other writers and
poets. Wordsworth performed the valuable service of gently pointing out to Hamilton that his talents did not lie in poetry: “You send me showers of verses which I receive with much pleasure . . . yet have we fears that this employment may seduce you from the path of science . . . I do venture to submit to your consideration, whether the poetical parts of your nature would not find a field more favorable to their nature in the regions of prose . . .”

Hamilton responded that his true poetry was his mathematics, and wisely turned to science. In 1827, while still an undergraduate, he was unanimously elected Professor of Astronomy at Trinity after the incumbent, John Brinkley, resigned to become Bishop of Cloyne. Hamilton started with a bang by publishing his book on optics—an entirely valid topic for an astronomer since it underlay the design of most astronomical instruments.

The link to mechanics was present only in embryonic form. The book's main focus, so to speak, was on the geometry of light rays—how they change direction when reflected in a mirror or refracted in a lens. “Ray optics” later gave way to “wave optics,” which recognized that light is a wave. Waves possess all sorts of extra properties, notably diffraction. Interference among waves can soften the edges of a projected image and even make light seem to bend around corners, a trick forbidden to rays.

The geometry of light rays was not a new topic; it had been studied extensively by earlier mathematicians, right back to Fermat, indeed, back to the Greek philosopher Aristotle. Now Hamilton did for optics what Legendre had famously done for mechanics: he got rid of the geometry and replaced it by algebra and analysis. Specifically, he replaced overt geometrical reasoning, based on diagrams, with symbolic calculations.

This was a major advance, because it replaced imprecise pictures with rigorous analysis. Later mathematicians made strenuous efforts to reverse Hamilton's path and reintroduce visual thinking. But by then, the formal algebraic stance had become part and parcel of mathematical thought, a natural companion to more overtly visual arguments. The wheel of fashion had come full circle but on a higher level, like a spiral staircase.