Map of a Nation (15 page)

Maskelyne’s achievement had significant repercussions for map-making. While he had been busy with his astronomical observations, his assistant Reuben Burrow made a meticulous survey of the mountain’s bulk. He and Maskelyne passed on the results of this survey to the Professor of Mathematics at the Royal Military Academy in Woolwich, Charles Hutton, who agreed to calculate the mountain’s volume. To do so, Hutton adopted a map-making technique that was a novelty in Britain. Contours trace the crinkles and furrows of landscape by joining up points of equal altitude on a hill or valley with a thin line. Otherwise known as isopleths, contours are now a defining feature of Ordnance Survey maps. It is thanks to these thin orange lines and their accompanying numbers that map-readers can conjure up a three-dimensional landscape in their minds; that hikers can brace themselves for the steep ascent around the next corner; and that the
promise
of a downward slope can be dangled before the tired members of a walking party. Contours had been used by Continental surveyors since the early eighteenth century, but British map-makers had traditionally tended to prefer a more pictorial mode of representation called hachuring: tiny
parallel
lines in black ink, whose density and darkness increased according to steepness (something similar had been used during the Military Survey of Scotland). Faced with the complex task of deducing Schiehallion’s volume,
Hutton produced a map on which points of equal altitude were linked by swirling lines. This was an important moment in cartographical history. Although contours would not become a standard component of
map-making
in Britain for another sixty years, Hutton’s pioneering use of the technique helped pave the way.

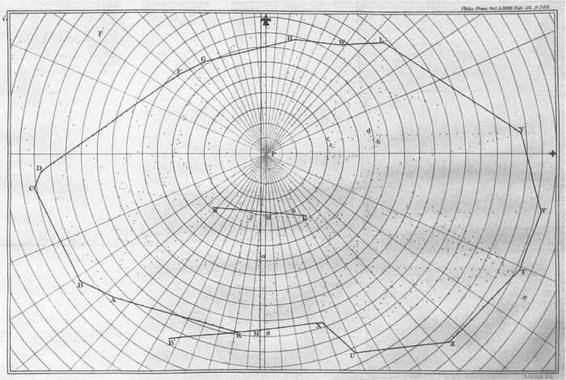
13. A simple contour map of Schiehallion by Charles Hutton, 1778.

 

The Schiehallion expedition was a vital landmark in Enlightenment attempts to find the earth’s concealed ‘pattern’ and to reveal the obscure ‘plan’ of its ‘mighty maze’ through empirical measurement. It also occurred at a point in William Roy’s life when it had become evident that he was as committed to scientific institutions and preoccupations as he was to military ones. In the decades that followed the Military Survey of Scotland, county surveyors had contributed fragmentary pieces towards a jigsaw map of the nation, military engineers had closely assessed the south coast of England for its vulnerability to invasion, and geodesists had measured portions of the earth to discover whether the planet’s shape bore out Newton’s laws. These events had taken place against a backdrop of frequent global wars and an apparent upsurge in nationalistic feeling in Britain. Institutions as diverse as the Society of Arts, the Board of Ordnance and the Royal Society had been the chief sponsors of cartographical progress in this period. Although William Roy had only possessed tangential links to the county map-makers of the Society of Arts’ competition, he had cut his teeth among the type of civilian estate-surveyors who proved its chief applicants, and he was deeply preoccupied with both military and geodetic traditions of map-making. His involvement in all these projects significantly strengthened his desire to put into place a national mapping agency.

 

 

 

C
HAPTER
T
HREE

 

‘L
E
M
INISTRE DES AFFAIRES
philosophiques’ was how a friend
half-jokingly
referred to Joseph Banks. He was not far wrong. By the early 1780s Banks’s magnetism and talents had elevated him to the most influential position in British science: President of the Royal Society. On the morning of 8 October 1783, we can imagine that he drew back his curtains with an eager anticipation of the day ahead. Only a month previously Britain had been released at last from conflict in the American War of Independence. Even the skies seemed to reflect a national feeling of release. All summer an ashy fog, the result of a volcanic eruption in southern Iceland, had filled the skies above Britain, dimming the stars and sun. By October the fog had lifted, the war was ended and the sun shone out as brightly as Banks’s mood.

It was a good day to receive the letter that was awaiting him on the
breakfast
table. The politician Charles James Fox had forwarded to Banks a ‘Mémoire’ that he had received from the director of the Paris Observatory, César François Cassini de Thury. This sought to persuade its reader that ‘il est interessant pour le progrés de l’Astronomie que l’on connaisse
exactement
la difference de Longitude et de Latitude entre les deux plus fameux observatoires de l’Europe’.
1
Cassini de Thury explained that, although the
exact longitude and latitude of the royal observatories at Paris and Greenwich were considered well known, in practice the English and French astronomers’ calculations of their longitudinal distance diverged to a worrying degree. He particularly called into question the determination of Greenwich’s situation. Banks did not have to be told that it was of the utmost importance that the positions of these sites were known with precision and confidence. Both observatories were essential to national and global navigation: for sailors, the problem that Cassini de Thury had raised could be a matter of life and death. Banks had no choice but to take his memorandum seriously.

César François Cassini de Thury was the third generation of a dynasty of astronomers who had presided over French cartography since 1669. In that year, a brilliant Italian star-gazer called Giovanni Domenico Cassini had moved to Paris and began to call himself ‘Jean Dominique Cassini’. He was granted membership of the newly founded institution for natural
philosophy
, the Académie Royale des Sciences, and in 1671 King Louis XIV gave Cassini a grant to establish the Paris Observatory, of which he became essentially director. By the end of the century Cassini had become involved in a project that had been initiated by the French astronomer Jean Picard to measure a meridian arc (a line travelling north–south across the earth’s surface) across the vertical length of France. This arc extended from Dunkirk in the north, through Paris, all the way down to the border with Spain, a little east of Perpignan, in south-west France. Picard and Cassini’s revelation of the country’s true extent was said to have provoked the King to comment that he had lost more land to his astronomers than to his enemies.

Cassini and Picard had measured their meridian arc using the most up-
to-date
technique of the day. Triangulation had first emerged as a map-making method in the mid sixteenth century when the Flemish mathematician Gemma Frisius set out the idea in his
Libellus de locorum describendorum ratione
(
Booklet concerning a way of describing places
), and by the turn of the eighteenth century it had become the most respected surveying technique in use. It was a similar method to plane-table surveying, but its instruments conducted measurements over much longer distances. The French meridian arc’s
triangulation
began when Cassini and Picard measured a baseline, a straight, level and unbroken line on a plain stretching from Villejuif, south of Paris,
seven miles further south to Juvisy (traversing the site of what is now the Orly airport). This baseline served as the cornerstone for the entire triangulation, and required rigorous and painstaking measurement, by laying measuring chains or sticks along the ground with great care. Cassini then positioned his theodolite exactly at one end of the baseline and trained its sights on a prominent nearby landmark, a church steeple or the top of a hill, for
example
, on which a colleague stood clutching a surveying staff, usually a flagstaff. Such targets in a triangulation are now known as ‘trig points’ or ‘trig
stations
’, which was an abbreviation of ‘trigonometrical’ and referred to the mathematical basis of the process. Once the theodolite was positioned so that the flagstaff filled the centre of its sights, Cassini stood back to read the angle between this sight line and the base line from the theodolite’s compass. He then repeated this process at the other end of the baseline, training the theodolite’s sights onto the same trig point and noting down the angle.

An imaginary triangle had thus been created, whose sides extended between the two ends of the baseline to the trig point. Cassini knew the length of the base from actual measurement, and with his theodolite he had discovered the two angles between the baseline and the two sight lines that stretched from its ends to the trig point. This one length and two angles were enough to allow Cassini to calculate the length of the other two sides of the triangle, using relatively simple trigonometric formulae. In doing so, he could discover the trig point’s distance from the baseline’s two ends. Cassini then repeated this process, using each side of this first triangle as the base for a new triangle that extended to a new trig point. Cassini replicated these
measurements
over and over again, building triangles on triangles, until a complex network of sight lines and measurements extended over the landscape.

Triangulation was extraordinarily useful because it discovered the relative distances and bearings of a series of landmarks scattered over a terrain, using sight alone. By ascertaining those lengths and angles, triangulation allowed the surveyor to calculate the longitude and latitude of the trig points, once the longitudinal and latitudinal positions of the baseline’s ends had been discovered through astronomical observations. Triangulation was a far quicker way of establishing large measurements than doing so with a chain on the ground, but it could be expensive, as it depended on instruments
sophisticated enough to precisely measure the angles of sight lines that extended over tens of miles and map-makers proficient in such observations and the complex mathematical equations that came afterwards.