Statistics Essentials For Dummies (47 page)

Finding the row, column, and grand totals
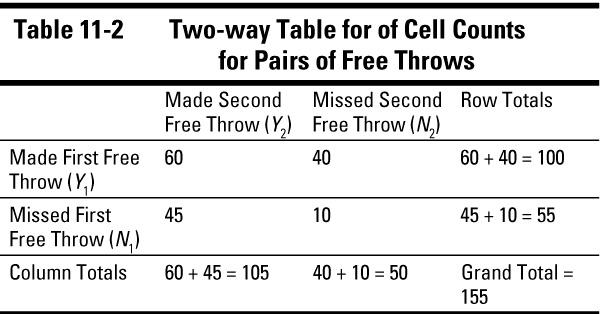
Once the cell counts are placed within a two-way table, it's always a good idea to total the rows and columns and write those totals in the margins. These are aptly named
marginal totals
. You can see in Table 11-2 that the total for the first row, 60 + 40 = 100, is written in the "Row Totals" column of the first row. This means the total number of times the first shot was successful was 100 (no matter what happened on the second shot). Similarly, the row total for row 2 represents the total number of times the first shot was missed regardless of what happened on the second shot (45 + 10 = 55).
The column totals are also included, listed in the row at the bottom of Table 11-2. The first column total is 60 + 45 = 105, which represents the total number of times a basket was made on the second shot (whether or not a basket was made on the first shot.) The second column total represents the total number of times the second shot was missed (regardless of what happened on the first shot), 40 + 10 = 50. Notice that the row totals sum to the grand total of 155, the total number of pairs of shots attempted. Similarly the column totals sum to the grand total of 155. The row, column, and grand totals are all shown in Table 11-2.
Finding Probabilities within a Two-Way Table
Once the two-way table is set up, you can begin using it to calculate probabilities and answer important questions about various events. For example, what is the probability that a player makes both free throws? That he makes the first one? That he makes the second free throw given that he misses the first one? And if he misses the first free throw, does that affect his chances of making the second one?
Figuring joint probabilities
A
joint probability
is the probability of the intersection of two outcomes or events. For example, the probability that a player makes the first free throw
and
the second free throw is a joint probability and is denoted P(
Y
1Y
2
). The word
and
provides a clue that it's a joint probability, as in "
A and B
."
Finding joint probabilities is easy using a two-way table, because the cells of the two-way table already show the number of individuals in each intersection. To find the probability of any intersection, take the number in that cell and divide by the grand total (found in the lower right corner of the two-way table). For example, the probability that a player makes the first free throw and the second, P(
Y
1Y
2
) is the number in the upper left cell of the two-way table, 60, divided by the grand total, 155. That means the probability of making both free throws is 60/155 = 0.39, or 39%.
The general formula for finding a joint probability using a two-
way table is
, where the cell in the
i
th
row
and the
j
th
column is denoted by cell (
i
,
j
).
Calculating marginal probabilities
A marginal probability is the probability of one outcome or event occurring, regardless of what happened with any other variables. For example, the probability that a player makes the first free throw (regardless of what happens on the second shot) is the marginal probability of
Y
1
and is denoted P(
Y
1
). And the probability that a player makes the second free throw (regardless of what happened on the first shot) is the marginal probability of
Y
2
, denoted P(
Y
2
).
To find the marginal probability of any single event, take the number in the corresponding row or column total and divide by the grand total. For example, look at the event that the player makes the first free throw (regardless of what happens on the second shot). This event is represented by row 1 of the two-way table, denoted
Y
1
(see Table 11-2). So the probability that a player makes the first free throw, P(
Y
1
), is found by taking the row 1 total, 100, and dividing by the grand total, 155, to get 0.65 or 65%.
Now look at the event that the player makes the second free throw. (Notice there is no mention of what happens on the first shot, so that tells you it's a marginal probability.) This event is represented in column 1 of the two-way table, denoted
Y
2
(see Table 11-2). So the probability that a player makes the second free throw, P(
Y
2
), is found by taking the column 1 total, 105, and dividing by the grand total, 155, to get 105/155 = 0.68 or 68%. So your first observation is true; he makes the second free throw more often than the first one (68% compared to 65%).
The general formula for finding the marginal probability of an
event in row
i
of the two-way table is P(row
i
event) =.
The general formula for finding the marginal probability of an event in column
j
of the two-way table is P(column
j
event) =
.
Finding conditional probabilities
A
conditional probability
is the probability of one event happening, given that the outcome of another event is known. For example, the probability that a player makes the second free throw, given that he made the first one, is the conditional probability of
Y
2
given
Y
1
and is denoted P(
Y
2
|
Y
1
). And the probability that a player misses the second free throw given that he missed the first one is the conditional probability of
N
2
given
N
1
and is denoted P(
N
2
|
N
1
).