Teaching the Common Core Math Standards With Hands-On Activities, Grades 3-5 (3 page)
Read Teaching the Common Core Math Standards With Hands-On Activities, Grades 3-5 Online
Authors: Judith A. Muschla,Gary Robert Muschla,Erin Muschla-Berry
Tags: #Education, #Teaching Methods & Materials, #Mathematics, #General

Activity: Combining Groups
Working in pairs or groups of three, students will generate ways that groups of items can be represented in real-world situations. They will then draw an illustration of the groups and write a description and a related multiplication sentence.
Materials
Drawing paper; crayons; colored pencils for each pair or group of students.
Procedure
1.
Ask your students to think about the ways things are grouped so that each group has the same number of items. Present the example of the hamburger rolls that was provided in the Background section. You may suggest other examples, such as sports (the number of starting players per team), board games (4 cards per person), shopping (6 cupcakes per package), school (5 books per student), and so on. Encourage your students to brainstorm other possible groups.
2.
Explain that students are to select an equal-sized group and then decide the number of groups they wish to represent. They are to draw a picture that illustrates their groups. For example, if they chose the packages of hamburger rolls, as noted in the Background section, they would draw 3 packages of hamburger rolls with 8 rolls per package.
3.
Explain that after they complete their drawings, they are to write a description of their groups and a multiplication sentence.
Closure
Discuss and display students' drawings, descriptions, and multiplication sentences.
Operations and Algebraic Thinking: 3.OA.2
“Represent and solve problems involving multiplication and division.”
2. “Interpret whole-number quotients of whole numbers, e.g., interpretas the number of objects in each share when 56 objects are partitioned equally into 8 shares, or as a number of shares when 56 objects are partitioned into equal shares of 8 objects each.”
Background
Division is the process of separating a quantity into equal groups. It is the inverse (opposite) of multiplication, which is the process of combining equal groups.
Activity: Breaking into Groups
Working in pairs or groups of three, students will find the number of groups that can be formed from a class of 30 students. They will represent their groups on graph paper.
Materials
Two to three sheets of graph paper; 30 counters for each pair or group of students.
Procedure
1.
Present this situation to your class: Mr. Smith has a class of 30 students. How many different-sized groups can he form?
2.
Explain that because Mr. Smith's class has 30 students, 1 counter represents 1 student.
3.
Instruct your students to divide their counters into equal groups to represent the students of Mr. Smith's class. They must find how many groups are possible and then sketch the groups on graph paper. Finally, have students write division sentences that represent their sketches.
Closure
Discuss your students' answers.
Answers
1 group of 30; 2 groups of 15; 3 groups of 10; 5 groups of 6; 6 groups of 5; 10 groups of 3; 15 groups of 2; 30 “groups” of 1
Operations and Algebraic Thinking: 3.OA.3
“Represent and solve problems involving multiplication and division.”
3. “Use multiplication and division within 100 to solve word problems in situations involving equal groups, arrays, and measurement quantities, e.g., by using drawings and equations with a symbol for the unknown number to represent the problem.”
Background
Diagrams and equations may be used with the operations of multiplication and division to solve word problems. Letters are commonly used to represent unknown numbers in equations.
Activity: It's a Match
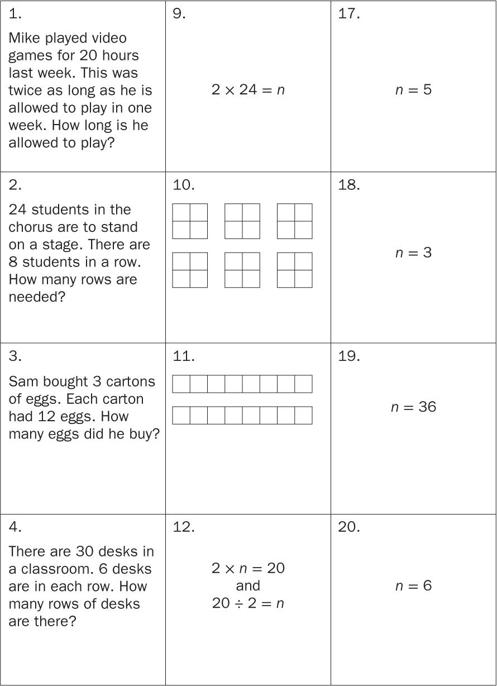
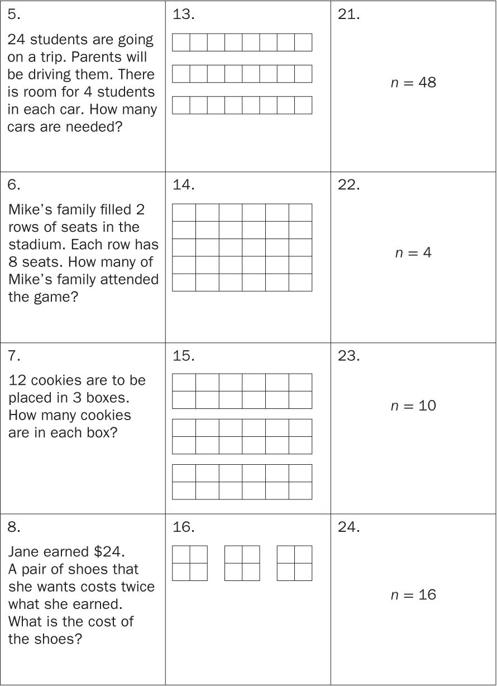
Working in groups, students will match word problems with equations, diagrams, and answers.
Materials
Scissors; one copy of reproducibles, “Matchings, I” and “Matchings, II,” for each group of students.
Procedure
1.
Explain that word problems involving multiplication and division can be solved by using equations or diagrams. In equations, symbols may be used to represent unknown numbers. For example, in the problemrepresents the product of
which is 15.
2.
Distribute copies of the reproducibles. Explain that together the reproducibles contain 24 boxes that have word problems (boxes 1–8), equations or diagrams (boxes 9–16), and answers (boxes 17–24).
3.
Explain that students are to cut out each box.
4.
Instruct students to start with problem 1. They should find the equation or diagram that matches the problem. Next they should find the answer that matches the problem. Students should continue in the same manner, matching equations, diagrams, and answers for problems 2, 3, and so on. They should place each set of correct “matchings” in separate piles.
Closure
Discuss students' results.
Answers
The card number of the problem, equation or diagram, and answer are listed in order: 1, 12, 23; 2, 13, 18; 3, 15, 19; 4, 14, 17; 5, 10, 20; 6, 11, 24; 7, 16, 22; 8, 9, 21
Matchings, I
Matchings, II
Operations and Algebraic Thinking: 3.OA.4