Grid of the Gods (58 page)

b. The Essential Imaginative Technique

Coxeter’s approach to higher dimensional geometry and its techniques was a profoundly intuitive and experiential one, notwithstanding the formal mathematics in which it is couched, relying upon the ability of the human imagination to grasp the basic conceptual principle on which the formal mathematical techniques were based. Typically, his intuitional experiential approach may be grasped in a series of short, pithy expressions that require deliberate thought to unpack. Indeed, Coxeter almost writes as an alchemical poet, crowding numerous layers of thought into extraordinarily short sentences.

The basic intuitive or experiential technique behind all the more formal principles and techniques of higher dimensional geometry may be glimpsed by the sentence that begins the seventh chapter of
Regular Polytopes
, “Ordinary Polytopes in Higher Space.” One may, at this point, be asking, “What
is
a ‘polytope’ anyway?” Coxeter answers the question, and in doing so, reveals the intuitive or imaginative technique in play: “
POLYTOPE
is the general term of the sequence
point, segment, polygon, polyhedron…”
¹⁵
Remember the “dimensional analysis” of the Pythagorean Tectratys of chapter 12? Coxeter is here saying the same thing: as one adds dimensions, one is able
to describe more and more things
, so that in zero dimensions, one can only describe a point, in one dimension, only a segment of a line, in two dimensions, polygons such as triangles, squares, octagons, and so on, and in three dimensions, polyhedra, such as tetrahedra, cubes, octrahedra, and so on. Coxeter puts this imaginative point more precisely as follows:

In space of no dimensions the only figure is a point, Πo. In space of one dimension we can have any number of point; two points bound a
line-segment
, Π1, which is the one-dimensional analogue of the polygon Π2 and polyhedron Π3. By joining Πo to another point, we construct Π1. By joining Π1 to a third point (outside its line) we construct a
triangle
, the simplest kind of Π2. By joining the triangle
to a fourth point (outside its plane) we construct a
tetrahedron
, the simplest Π3. By joining the tetrahedron to a fifth point (outside its 3-space!) we contruct a
pentatope
, the simplest Π4… The general case is now evident: any
n
+1 points which do not lie in an (n-1)- space are the vertices of an
n
-dimensional
simplex…
¹⁶

The term “polytopes” (Π) thus denotes
what happens to polyhedra when they are rotated into more than three dimensions.
Or to put it differently, the term “polytope” denotes the common mathematical elements of the same figure in various dimensions, such as the progression in the above quotation from Coxeter, of “triangle, teatrahedron, pentatope” and so on. Notice also, that in each of the two previous examples — polygons in two dimensions and polyhedra in three — that the shapes of the polyhedra result from performing what geometers call an “orthorotation,” or a rotation from two dimensions into a third dimension that is perpendicular or at 90 degree angles from the other two. The same thing happens in the rotation from three to four, and from four to five dimensions: each dimensional orthorotation simply adds another spatial dimension to the previous ones, in a dimension at right angles to all the previous dimensions.

Obviously, this cannot exist in a three dimensional space any more than a three dimensional polyhedron can exist in a two dimensional space, but they can be
pictured
or represented in a two dimensional space, and more importantly, they can be exactly mathematically described by certain specific techniques that we will get to in a moment. To put Coexter’s point about polytopes of certain types being analogues — there’s that crucial word again! — somewhat differently,
all polytopes
of a specific type — say, triangles, tetrahedra, and pentatopes — share certain properties in common
regardless
of how many dimensions in which they occur;
they are, in “physics terms,” all coupled harmonic oscillators to one another across dimensions, and the “coupling” occurs in the numbers themselves, numbers that in turn result from the regular geometry of their shape.
To boil it all down, all polytopes of a particular species have a “shape” in n-dimensions, and that shape is
projected into the dimensional space in which it exists — say, two, three, or four dimensions — by certain regular mathematical laws.

Now let us observe yet another implication of Coxeter’s always deceptively simple remarks, namely, that
the simplest way to describe the orthorotation of any polytope or “shape” from one type of dimensional space to another is tetrahed rally
. Coxeter puts this point with his customarily deceptively simple statements: “Two-dimensional polytopes are merely polygons… Three-dimensional polytopes are polyhedra…”
¹⁷

A “triangular” shape in two dimensions is a natural oscillator of the same shape in three, four, and more dimensions, and
this
is the real encoded secret in the Sacred Tectratys and Pentactys of the Pythagoreans, for the simple symbol is also a symbol of this mathematical technique, yet another legacy from High Antiquity, for it is essential to recall that the Pyragoreans regarded it as a key cosmological secret:

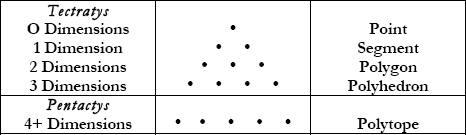
The Pythagoreans, in other words, were preserving a secret of hyper-dimensional geometry, whether they knew it or not, and given all the indications that they knew the image concealed a great a various multi-leveled meaning, they may, indeed, have had an inkling of it.

c. The Essential Mathematical Techniques

With these things in mind, we are ready to address the next question: what are the
exact
mathematical techniques used to describe
polytopes in more than three, or >3, dimensions? Coxeter begins
Regular Polytopes
by describing one such technique in detail:

To be precise, we define a
p
-gon as a circuit of
p
line-segments A
₁
A
₂
, A
₂
A
₃
,…, A
_p
A
₁
, joining consecutive pairs of
p
points A
₁
, A
₂
, …, A
_p
. The segments and points are called
sides
and
vertices
. Until we come to Chapter VI we shall insist that the sides do not cross one another. If the vertices are all coplanar we speak of a
plane
polygon, otherwise a
skew
polygon.

A plane polygon decomposes its plane into two regions…

(Remember our topological metaphor, and that initial differentiation of the dimensionless Nothing into two regions, joined upon a common surface? Coexter is now describing
the same process
in
two
dimensions, via a process of differentiation of a two dimensional Nothing, a “plane”, by means of a regular polygon whose surface joins its finite interior with its infinite exterior! In other words, you have been doing higher-dimensional geometry all along, in the topological metaphor, the only difference between topology and geometry being, that topology is not dealing with geometrical objects, but the spaces themselves! To return to Coxeter):

…

A plane polygon decomposes its plane into two regions, one of which, called the
interior
, is finite. We shall often find it convenient to regard the
p
-gon as consisting of its interior as well as its sides and vertices. We can then re-define it as a simply-connected region bounded by
p
distinct segments.
¹⁸

Before we distill all this
lingua mathematica arcana
, there is one more statement, again from the beginning of the book, worth citing:

A polyhedron may be defined as a finite, connected set of plane polygons
,
such that every side of each polygon belongs also to just one other polygon
, with the proviso that the polygons surrounding each vertex form a single circuit(to exclude anomalies such as two pyramids with a common apex). The polygons are called
faces
, and their edges
sides
. Until Chapter VI we insist that the faces do not cross one another. Thus
the polyhedron forms a single closed surface, and
decomposes (three dimensional)
¹⁹
space into two regions, one of which, called the interior, is finite.
²⁰

Again, Coxeter has described a process of three-dimensional differentiation of a three-dimensional space by means of a polyhedron.

So what is the first basic mathematical principle in evidence in the transition of any polytope from one system of dimensional spaces to another?

It’s so simple that you, the reader,
know it already
, and have known it since elementary school:
one counts the

1) points, or vertices of an object;

2) the edges, or
lines
of an object; and

3) the
faces
of an object.

Thus, a triangle has three vertices, three edges, and one “face”; a teatrahedron four vertices, six edges, and four faces, and so on. A square has four vertices, four edges, and one “face”; a cube has eight vertices, twelve edges, and six faces, and so on.

To this technique there is added yet another, and this one is a bit more complicated, but it is also the crucial technique. We begin, once again, with Coxeter’s own summation of this technique:

A regular polygon is easily seen to have a
centre
, from which all the vertices are at the same distance o
R
, while all the sides are at the same distance 1R. This means that there are
two concentric circles, the circum-circle and in-circle
, which pass through the vertices and touch the sides, respectively.

And, notes Coxeter in the very next sentence, anticipating a physics application:

It is sometimes helpful to think of the side of a
p
-gon as representing
p
vectors whose sum is zero.
²¹

In other words, for any regular polygon, such as a square, it is possible to draw a circle whose center shares the center of the square, and whose circumference touches upon, or is tangent to, the four vertices of the square, which is the circumscribing circle, and it is also possible to draw a circle whose circumference touches upon, or is tangent to, the
edges
of the square, which is the circuminscribed circle.

But note that Coxeter is describing a process that, like the polygon itself, can be orthorotated into three or more dimensions, in which case, the circuminscribing and circumscribed circles, become cicuminscribing and circuminscribed
spheres,
and
hyper-
spheres. But the
numbers
will be preserved in all dimensional spaces.

Now we consider the next most difficult component of these circumscribing and circumscribed
n
-circles.
²²
If we imagine a circle in two dimensions, and a square within it, obviously the square will touch on four points of a circumscribing circle. If we now instead circuminscribe an octagon, there will be eight points on touching on the circle. Dividing the octagon again will produce sixteen touching points, and with each such division, the regular polygon assumes a shape closer and closer to the circuminscribing circle. Similarly a process in three dimensions with regular polyhedra will more and more approximate the shape of the circuminscribing sphere. Notably, the circumference of the circle is, as everyone knows, C=2πr, where C is the circumference and r is the radius of any given circle. Thus, as the regular polygons circumscribed in a circle more closely approximate the circle itself, the closer they get to that crucial relationship of 2πr. In other words, the relationship of 2π and its multiples becomes a crucial component of rotations into more than three dimensions.
²³

This point about “squaring the circle” is also quite an important technique for this type of higher dimensional mathematical technique, for it allows geometers to determine the numerical relationships of objects in more than three dimensions to their circuminscribing and circuminscribed hyper-spheres. It is, along with the counting of vertices, edges, and faces (in order to determine what
type
of object one is dealing with), the essential technique.