Out of My Later Years: The Scientist, Philosopher, and Man Portrayed Through His Own Words (15 page)

In an early stage the words may correspond directly to impressions. At a later stage this direct connection
is
lost insofar as some words convey relations to perceptions only if used in connection with other words (for instance such words as: “is,” “or,” “thing”). Then word-groups rather than single words refer to perceptions. When language becomes thus partially independent from the background of impressions a greater inner coherence is gained.

Only at this further development where frequent use is made of so-called abstract concepts, language becomes an instrument of reasoning in the true sense of the word. But it is also this development which turns language into a dangerous source of error and deception. Everything depends on the degree to which words and word-combinations correspond to the world of impression.

What is it that brings about such an intimate connection between language and thinking? Is there no thinking without the use of language, namely in concepts and concept-combinations for which words need not necessarily come to mind? Has not everyone of us struggled for words although the connection between “things” was already clear?

We might be inclined to attribute to the act of thinking complete independence from language if the individual formed or were able to form his concepts without the verbal guidance of his environment. Yet most likely the mental shape of an individual, growing up under such conditions, would be very poor. Thus we may conclude that the mental development of the individual and his way of forming concepts depend to a high degree upon language. This makes us realize to what extent the same language means the same mentality. In this sense thinking and language are linked together.

What distinguishes the language of science from language as we ordinarily understand the word? How is it that scientific language is international? What science strives for is an utmost acuteness and clarity of concepts as regards their mutual relation and their correspondence to sensory data. As an illustration let us take the language of Euclidian geometry and Algebra. They manipulate with a small number of independently introduced concepts, respectively symbols, such as the integral number, the straight line, the point, as well as with signs which designate the fundamental operations, that is the connections between those fundamental concepts. This is the basis for the construction, respectively definition of all other statements and concepts. The connection between concepts and statements on the one hand and the sensory data on the other hand is established through acts of counting and measuring whose performance is sufficiently well determined.

The super-national character of scientific concepts and scientific language is due to the fact that they have been set up by the best brains of all countries and all times. In solitude and yet in cooperative effort as regards the final effect they created the spiritual tools for the technical revolutions which have transformed the life of mankind in the last centuries. Their system of concepts have served as a guide in the bewildering chaos of perceptions so that we learned to grasp general truths from particular observations.

What hopes and fears does the scientific method imply for mankind? I do not think that this is the right way to put the question. Whatever this tool in the hand of man will produce depends entirely on the nature of the goals alive in this mankind. Once these goals exist, the scientific method furnishes means to realize them. Yet it cannot furnish the very goals. The scientific method itself would not have led anywhere, it would not even have been born without a passionate striving for clear understanding.

Perfections of means and confusion of goals seem—in my opinion—to characterize our age. If we desire sincerely and passionately the safety, the welfare and the free development of the talents of all men, we shall not be in want of the means to approach such a state. Even if only a small part of mankind strives for such goals, their superiority will prove itself in the long run.

16

The Laws of Science and the Laws of Ethics

SCIENCE SEARCHES FOR RELATIONS which are thought to exist independently of the searching individual. This includes the case where man himself is the subject. Or the subject of scientific statements may be concepts created by ourselves, as in mathematics. Such concepts are not necessarily supposed to correspond to any objects in the outside world. However, all scientific statements and laws have one characteristic in common: they are “true or false” (adequate or inadequate). Roughly speaking, our reaction to them is “yes” or “no.”

The scientific way of thinking has a further characteristic. The concepts which it uses to build up its coherent systems are not expressing emotions. For the scientist, there is only “being,” but no wishing, no valuing, no good, no evil; no goal. As long as we remain within the realm of science proper, we can never meet with a sentence of the type: “Thou shalt not lie.” There is something like a Puritan’s restraint in the scientist who seeks truth: he keeps away from everything voluntaristic or emotional. Incidentally, this trait is the result of a slow development, peculiar to modern Western thought.

From this it might seem as if logical thinking were irrelevant for ethics. Scientific statements of facts and relations, indeed, cannot produce ethical directives. However, ethical directives can be made rational and coherent by logical thinking and empirical knowledge. If we can agree on some fundamental ethical propositions, then other ethical propositions can be derived from them, provided that the original premises are stated with sufficient precision. Such ethical premises play a similar role in ethics, to that played by axioms in mathematics.

This is why we do not feel at all that it is meaningless to ask such questions as: “Why should we not lie?” We feel that such questions are meaningful because in all discussions of this kind some ethical premises are tacitly taken for granted. We then feel satisfied when we succeed in tracing back the ethical directive in question to these basic premises. In the case of lying this might perhaps be done in some way such as this: Lying destroys confidence in the statements of other people. Without such confidence, social cooperation is made impossible or at least difficult. Such cooperation, however, is essential to make human life possible and tolerable. This means that the rule “Thou shalt not lie” has been traced back to the demands: “Human life shall be preserved” and “Pain and sorrow shall be lessened as much as possible.”

But what is the origin of such ethical axioms? Are they arbitrary? Are they based on mere authority? Do they stem from experiences of men and are they conditioned indirectly by such experiences?

For pure logic all axioms are arbitrary, including the axioms of ethics. But they are by no means arbitrary from a psychological and genetic point of view. They are derived from our inborn tendencies to avoid pain and annihilation, and from the accumulated emotional reaction of individuals to the behavior of their neighbors.

It is the privilege of man’s moral genius, impersonated by inspired individuals, to advance ethical axioms which are so comprehensive and so well founded that men will accept them as grounded in the vast mass of their individual emotional experiences. Ethical axioms are found and tested not very differently from the axioms of science. Truth is what stands the test of experience.

17

An Elementary Derivation of the Equivalence of Mass and Energy

THE FOLLOWING DERIVATION of the law of equivalence, which has not been published before, has two advantages. Although it makes use of the principle of special relativity, it does not presume the formal machinery of the theory but uses only three previously known laws:

(1) The law of the conservation of momentum.

(2) The expression for the pressure of radiation; that is, the momentum of a complex of radiation moving in a fixed direction.

(3) The well known expression for the aberration of light (influence of the motion of the earth on the apparent location of the fixed stars—Bradley).

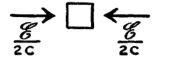
We now consider the following system. Let the body B rest

freely in space with respect to the system K
₀
. Two complexes of radiation S, S
′
each of energy
E/2
move in the positive and negative x
₀
direction respectively and are eventually absorbed by B. With this absorption the energy of B increases by E. The body B stays at rest with respect to K
₀
by reasons of symmetry.

Now we consider this same process with respect to the system K, which moves with respect to K
₀
with the constant velocity v in the negative Z
₀
direction. With respect to K the description of the process is as follows:

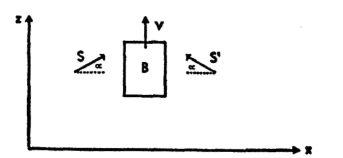
The body B moves in the positive Z direction with velocity v. The two complexes of radiation now have directions with respect to K which make an angle α with the x axis. The law of aberration states that in the first approximation α = c/v, where c is the velocity of light. From the consideration with respect to K
_o
we know that the velocity v of B remains unchanged by the absorption of S and S
′
.

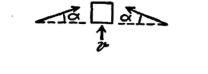
Now we apply the law of conservation of momentum with respect to the z direction to our system in the coordinate-frame K.

I.
Before the absorption
let M be the mass of B; Mv is then the expression of the momentum of B (according to classical mechanics). Each of the complexes has the energy E/2 and hence, by a well known conclusion of Maxwell’s theory, it has the momentum E/2c. Rigorously speaking this is the momentum of S with respect to K
_o
. However, when v is small with respect to c, the momentum with respect to K is the same except for a quantity of second order of magnitude (v
₂
/c
₂
compared to 1). The z-component of this momentum is E/2c sin α or with sufficient accuracy (except for quantities of higher order of magnitude) E/2c α or E/2c. v/c
²
. S and S′ together therefore have a momentum E v/c
²
in the z direction. The total momentum of the system before absorption is therefore