Collected Essays (57 page)

I used to have the mistaken idea that a tree branched by splitting the tips of its branches, but this isn’t really the way it works. The way that real trees grow is that a new branch forms upon the smooth part of any sufficiently long piece. That is, it’s useful to think of “side-branching” trees rather than “tip-branching” trees. Below is four steps in the construction of a so-called Tokunga tree which actually ends up with a dimension of 2, that is, it fills up space.

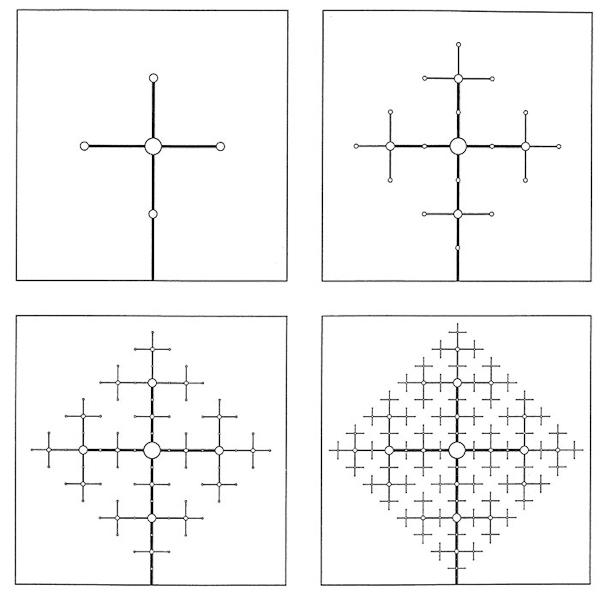
A branching tree, a fractal of dimension 1.46.

River drainage basins approach being side-branching Tokunga trees, as are the blood vessels in your body or the veins in leaf. Side-branching trees manage to fill up all the space available to them.

You might object to my calling a physical object like a leaf or an oak tree a fractal, because, for instance, your oak’s branching structure does not in fact have endlessly many levels of detail (as a true mathematical fractal would). When you get down to the twig level, the parts no longer resemble the whole. No matter. Even though an actual physical tree has a limited number of branching levels, it can be useful to think of it being a fractal. What we’re doing here is a special kind of idealization in which we approximates high complexity by infinite complexity. Oddly enough, this makes things easier. As the mathematician Stan Ulam once said about a particular problem, “The infinite case is easy. The finite case takes too long.”

Alright, now I’m ready to state my point. Both the Web and the mental world of your ideas are side-branching N-dimensional fractal trees.

The Web and the Mind Are Fractals

In the first part of this essay, I said that the Web might be a good model for the human mind. I said that one reason for this is that both the Web and the mind are like fractals. And then I spent the rest of the column explaining what a fractal is. Now let’s why the Web and the mind are indeed similar kinds of fractals.

There is a loose sense in which thinking is like moving about in a space of ideas. I visit this notion or emotion, then that one, and then perhaps I return to the first thought. My familiar thoughts are somewhat fixed and persistent, a bit like objects in a landscape. Suppose that I use the word “mindscape” to stand for the manifold of possible thoughts.

There’s clearly some overlap between my mindscape and yours. It’s suggestive to imagine that our mindscapes are really just different views of one Platonic super-mindscape. It’s like we’re in different rooms in a big town looking at the city outside. Though it’s hard for us to see the stuff hidden in each others’ rooms, when we look out our windows we pretty much the same collection of streets, buildings, clouds, mountains, pedestrians and so on. And if you can’t see a particular mindscape sight from where you are, I can tell you a way to get there.

But looking at the mindscape really isn’t very much like looking out a window after all. Things change, and split, and melt together. Each thought sets off fireworks of associations that in turn lead to further thoughts. You start out thinking about a soda, and the next thing you know you’re thinking about tap-dancing.

One of the reasons I love writing is because language is itself so slippery and fractal. Many words and phrases have the peculiar property of meaning, or at least suggesting, several different things. And a given passage of text can sometimes be interpreted at several levels.

Language evolved both to describe the world around us, but also as a way for people to represent the contents of their own minds. “What are you thinking?” “Well, let me tell you.”

Just like the mind, language itself has a branching quality. Suppose, for instance, you were to make a diagram with a word at each node. And now suppose you drew a line from each word to every other word that appears in, say, the standard dictionary definition of the first word. What a mess! Everything’s stuck to everything else.

Earlier I talked about a kind of fractal curve (the Koch curve) where a new bump buds out of the middle of every line. We can see this kind of thing happening in thought as follows.

Suppose I say that A (soda) reminds me of B (tap-dancing). Then I have a node A, a node B, and a line between them. But now you ask me about why A reminds me of B, I form a bump C, which holds a concept having to do with the connecting branch. C might be, for instance, the Rockettes.

Soda reminds me of tap-dancing because of the Rockettes. What could be more obvious? Obvious to me, but not to you! So now I make the bump bumpier. I’ve got an image of myself as a twelve-year-old boy at Radio City Music Hall drinking a Pepsi (sponsor product placement!) watching the Rockettes. Fine. But there’s another bump upon this. I’ve never been inside Radio City Music Hall. It was my boyhood friend Niles who went there, and he told me about it so vividly that I felt like I’d seen it myself. So now I better tell you about Niles and me back in 1950s Louisville…

A and B lead to C, D, E, and on beyond Z.

Another example. The figure below shows a sketch of a language net that I drew in Virginia in 1987. It starts from the sentence, “I picked up a lit cigarette from the ashtray to the right of the keyboard and took a puff.”

Figure 17: A fractal association net in my mindscape of 1987.

Although Figure 17 is drawn in the form of a branching tree, one should really think of each of the link lines as itself having further lines come off of it. The replacement step I have in mind appears in Figure 18.

Figure 18: The way the branching “actually” works.

If we were to repeatedly perform the step in Figure 18, we’d be heading towards something like the Peano curve. But it seems that the successive levels of detail would overlap each other. One solution to this would be to imagine embedding the image up into a higher-dimensional space. In particular, if I were to have replace each pair of related ideas by 2
^N
ideas, I’d end up with a fractal of dimension N.

We need to make two disclaimers when we speak of “real” physical or mental objects as fractals. First of all, these objects are perhaps not infinitely complex. And secondly, these objects are irregular fractals.

Regarding the first disclaimer, infinity comes naturally to mathematicians, and pure mathematical fractals are thought of as having endlessly many levels of detail. One might think, for instance, of a tree each of whose branch segments has subbranches coming off of it, with the subbranches having subsubbranches coming off them, and so on forever. But even though an actual physical tree is likely to have only some five to seven levels of branching, it’s sometimes useful to think of it being a fractal. This kind of mathematical idealization approximates a large complexity by infinite complexity. Oddly enough, for a mathematician, infinity is often easier to handle than some very large number N. This kind of approximation is kindred to the opposite kind of approximation, in which we replace complexity by simplicity, as when we think of a planet or a star as a sphere, or even as a point.

Figure 19: A random fractal.

Regarding the second disclaimer, the parts of even a pure mathematical fractal need not be perfect copies of the whole. It’s perfectly all right to have the patterns vary a bit from level to level. Thus a hillock on a mountain looks quite a bit like the whole mountain, but it isn’t an exact replica. The boulders on the hillock in turn resemble the hillock, but they aren’t scale models of it. And so on. Figure 19 shows how we might construct a random mathematical fractal with a dimension of about 1.1. (By the way, with irregular fractals like this we can’t use the simple “P and Q” rule for calculating the dimension, but there is an alternate method.)

Recall now that the purpose of my talk is to argue that the Web is in some respects like the human mind. So now I need only to point out that the Web does indeed have a fractal quality to it. One starts out headed for topic A, then finds a link to topic B, gets distracted by a connection to topic C, and so on, once again all the way beyond Z.

As it turns out there are many different kinds of fractals, so we might well ask which kind of fractal might best serve as a model for the Mind. The Koch curve in particular is not so well-fitting a model for the web as is a tree or a cloud.

As was discussed above, you get a tree by repeatedly shooting new branches off the old branches, and this is a little like the way web-links (and mental associations) form. Viewed as a geometrical structure, this kind of tree is hard to draw, for if you try and draw a densely branching tree on a piece of paper, you quickly run out of room. Some of the lines end up crossing over the other lines. (To fit the extra lines in we can either ask for more room or we can bump our drawing up into higher dimensions.) But it’s easy to imagine such a tree. And the Web lends itself to representing a highly branching mind-tree because we can stick in as many links as we want.

But before committing to the idea of a tree, let’s think a bit about clouds. When I say that a cloud is a fractal, I have in mind a model in which we think of a cloud as a certain shaded volume of space. This shaded cloud region has a very complicated shape, with lots of holes and tendrils. One way to imagine mathematically constructing a cloud is to start with a cube of space and to then subdivide it into, say, twenty-seven subcubes (cutting it in three along each dimension, like a Rubik’s cube.). Remove each subcube that doesn’t have any of the cloud in it. Then take each of the remaining subcubes and divide it into twenty-seven subsubcubes. Again remove the pieces that don’t touch the cloud. Repeat the process of dividing and winnowing out for a number of levels. If done in a regular fashion this can lead to a regular fractal such as the “Menger Sponge.”

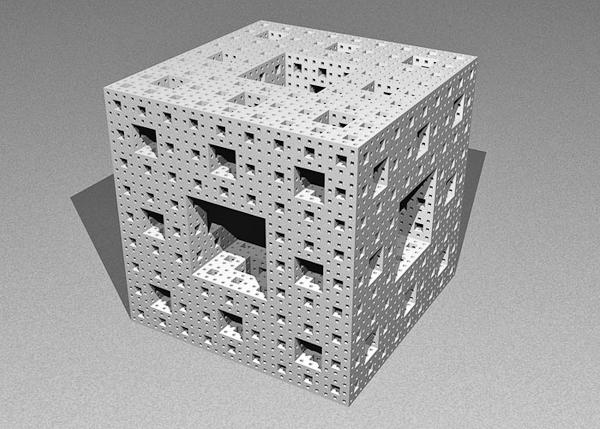
The Menger Sponge (image from Wikipedia).

Of course you don’t have to build clouds up in such a regular way. You can use a more random process for removing subcubes, and then you end up with something more natural in appearance.

Maybe a mind is as much like a cloud as it is like a tree. You have some vague notion (like a cloud seen from a distance), and then when you examine it more closely it breaks into a number of denser regions. And these chunks in turn break into smaller chunks.

We’ve been talking about the mind being a fractal, and the web is a fractal too. Cruising the web, one starts out headed for topic A, but when you get to the page for A, you notice a link to topic B, and you go look at B before reading A, but on page B, you find a tempting link C that you just have to read first, and so on.

In some sense you never can get started drawing a true fractal like the Koch curve, because you always have to put in another bump before the bump you want to get to. This is similar to the experience you have when you try to fully explain any aspect of your mindscape. And this is an experience you can also have when you surf the Web.

The attractive thing about the Web as a model of the mind is that its a kind of “paper” where you never have to “run off the edge” or “run out of dimensions.” You can always add scrollbars or links to give yourself more room.

Certainly at this point in history, the Web doesn’t match the branching-tree structure of a real human mind, but a Web-like structure
could be tuned
to be a tree like this.

Or, again, if we want to think of the mind as being like a cloud, we can also think of a web page as being like a cloud. It’s a collection of concepts, and many of these can be hyperlinked to further web pages.

In other words, we can either think of a web page as branching like a tree or as having denser regions like a cloud.

So the mind and the web both have fractal qualities. Does this mean the web can be a good model for the mind? Whenever I discuss this idea with people I get a lot of objections. Here are a few of them, with my attempts an answers.

Objection 1
. Just because the Web and the Mind are like fractals doesn’t mean they’re like each other. A Koch curve, a tree and a cloud are fractals, but they aren’t the same.

Answer 1
. The Web is endlessly tunable. I’m not saying that the Web
right now
is like the Mind. I’m saying that it should be possible to use the Web to make a good representation of a mind.