A Briefer History of Time (10 page)


Smeared Quantum Position
According to quantum theory, one cannot pinpoint an object’s position and velocity with infinite precision, nor can one predict exactly the course of future events.
The test of a scientific theory, as we have said, is its ability to predict the results of an experiment. Quantum theory limits our abilities. Does this mean quantum theory limits science? If science is to progress, the way we carry it on must be dictated by nature. In this case, nature requires that we redefine what we mean by prediction: We may not be able to predict the outcome of an experiment exactly, but we can repeat the experiment many times and confirm that the various possible outcomes occur within the probabilities predicted by quantum theory. Despite the uncertainty principle, therefore, there is no need to give up on the belief in a world governed by physical law. In tact, in the end, most scientists were willing to accept quantum mechanics precisely because it agreed perfectly with experiment.
One of the most important implications of Heisenberg’s uncertainty principle is that particles behave in some respects like waves. As we have seen, they do not have a definite position but are "smeared out" with a certain probability distribution. Equally, although light is made up of waves, Planck’s quantum hypothesis also tells us that in some ways light behaves as if it were composed of particles: it can be emitted or absorbed only in packets, or quanta. In fact, the theory of quantum mechanics is based on an entirely new type of mathematics that no longer describes the real world in terms of either particles or waves. For some purposes it is helpful to think of particles as waves and for other purposes it is better to think of waves as particles, but these ways of thinking are just conveniences. This is what physicists mean when they say there is a duality between waves and particles in quantum mechanics.
An important consequence of wavelike behavior in quantum mechanics is that one can observe what is called interference between two sets of particles. Normally, interference is thought of as a phenomenon of waves; that is to say, when waves collide, the crests of one set of waves may coincide with the troughs of the other set, in which case the waves are said to be out of phase. If that happens, the two sets of waves then cancel each other out, rather than adding up to a stronger wave, as one might expect. A familiar example of interference in the case of light is the colors that are often seen in soap bubbles. These are caused by reflection of light from the two sides of the thin film of water forming the bubble. White light consists of light waves of all different wavelengths, or colors. For certain wavelengths the crests of the waves reflected from one side of the soap film coincide with the troughs reflected from the other side. The colors corresponding to these wavelengths are absent from the reflected light, which therefore appears to be colored.

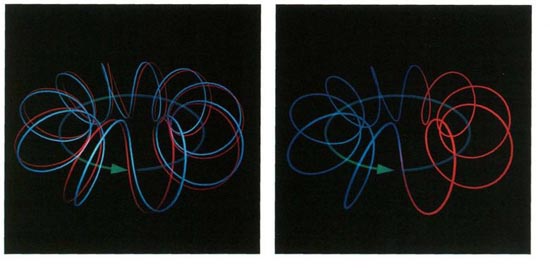
In and Out of Phase
If the crests and troughs of two waves coincide, they result in a stronger wave, but if one wave’s crests coincide with another’s troughs, the two waves cancel each other.
But quantum theory tells us that interference can also occur for particles, because of the duality introduced by quantum mechanics. A famous example is the so-called two-slit experiment. Imagine a partition—a thin wall—with two narrow parallel slits in it. Before we consider what happens when particles are sent through these slits, let’s examine what happens when light is shined on them. On one side of the partition you place a source of light of a particular color (that is, of a particular wavelength). Most of the light will hit the partition, but a small amount will go through the slits. Now suppose you place a screen on the far side of the partition from the light. Any point on that screen will receive waves from both slits. However, in general, the distance the light has to travel from the light source to the point via one of the slits will be different than for the light traveling via the other slit. Since the distance traveled differs, the waves from the two slits will not be in phase with each other when they arrive at the point. In some places the troughs from one wave will coincide with the crests from the other, and the waves will cancel each other out; in other places the crests and troughs will coincide, and the waves will reinforce each other; and in most places the situation will be somewhere in between. The result is a characteristic pattern of light and dark.
The remarkable thing is that you get exactly the same kind of pattern if you replace the source of light by a source of particles, such as electrons, that have a definite speed. (According to quantum theory, if the electrons have a definite speed the corresponding matter waves have a definite wavelength.) Suppose you have only one slit and start firing electrons at the partition. Most of the electrons will be stopped by the partition, but some will go through the slit and make it to the screen on the other side. It might seem logical to assume that opening a second slit in the partition would simply increase the number of electrons hitting each point of the screen. But if you open the second slit, the number of electrons hitting the screen increases at some points and decreases at others, just as if the electrons were interfering as waves do, rather than acting as particles. (See illustration on page 97.)

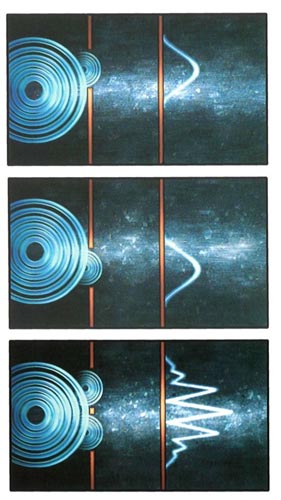
Path Distances and Interference
In the two-silt experiment, the distance that waves must travel from the top and bottom slits to the screen varies with height along the screen The result is that the waves reinforce each other at certain heights and cancel at others, forming an interference pattern
Electron Interference
Because of interference, the result of sending a beam of electrons through two slits does not correspond to the result of sending the electrons through each slit separately.
Now imagine sending the electrons through the slits one at a time. Is there still interference? One might expect each electron to pass through one slit or the other, doing away with the interference pattern. In reality, however, even when the electrons are sent through one at a time, the interference pattern still appears. Each electron, therefore, must be passing through both slits at the same time and interfering with itself!
The phenomenon of interference between particles has been crucial to our understanding of the structure of atoms, the basic units out of which we, and everything around us, are made. In the early twentieth century it was thought that atoms were rather like the planets orbiting the sun, with electrons (particles of negative electricity) orbiting around a central nucleus, which carried positive electricity. The attraction between the positive and negative electricity was supposed to keep the electrons in their orbits in the same way that the gravitational attraction between the sun and the planets keeps the planets in their orbits. The trouble with this was that the classical laws of mechanics and electricity, before quantum mechanics, predicted that electrons orbiting in this manner would give off radiation. This would cause them to lose energy and thus spiral inward until they collided with the nucleus. This would mean that the atom, and indeed all matter, should rapidly collapse to a state of very high density, which obviously doesn’t happen!
The Danish scientist Niels Bohr found a partial solution to this problem in 1913. He suggested that perhaps the electrons were not able to orbit at just am distance from the central nucleus but rather could orbit only at certain specified distances. Supposing that only one or two electrons could orbit at any one of these specified distances would solve the problem of the collapse, because once the limited number of inner orbits was full, the electrons could not spiral in any farther. This model explained quite well the structure of the simplest atom, hydrogen, which has only one electron orbiting around the nucleus. But it was not clear how to extend this model to more complicated atoms. Moreover, the idea of a limited set of allowed orbits seemed like a mere Band-Aid. It was a trick that worked mathematically, but no one knew why nature should behave that way, or what deeper law—if any—it represented. The new theory of quantum mechanics resolved this difficulty. It revealed that an electron orbiting around the nucleus could be thought of as a wave, with a wavelength that depended on its velocity. Imagine the wave circling the nucleus at specified distances, as Bohr had postulated. For certain orbits, the circumference of the orbit would correspond to a whole number (as opposed to a fractional number) of wavelengths of the electron. For these orbits the wave crest would be in the same position each time round, so the waves would reinforce each other. These orbits would correspond to Bohr’s allowed orbits. However, for orbits whose lengths were not a whole number of wavelengths, each wave crest would eventually be canceled out by a trough as the electrons went round. These orbits would not be allowed. Bohr’s law of allowed and forbidden orbits now had an explanation.
A nice way of visualizing the wave/particle duality is the so-called sum over histories introduced by the American scientist Richard Feynman. In this approach a particle is not supposed to have a single history or path in space-time, as it would in a classical, nonquantum theory. Instead it is supposed to go from point A to point B by every possible path. With each path between A and B, Feynman associated a couple of numbers. One represents the amplitude, or size, of a wave. The other represents the phase, or position in the cycle (that is, whether it is at a crest or a trough or somewhere in between). The probability of a particle going from A to B is found by adding up the waves for all the paths connecting A and B. In general, if one compares a set of neighboring paths, the phases or positions in the cycle will differ greatly. This means that the waves associated with these paths will almost exactly cancel each other out. However, for some sets of neighboring paths the phase will not vary much between paths, and the waves for these paths will not cancel out. Such paths correspond to Bohr’s allowed orbits.