When Computers Were Human (22 page)
Read When Computers Were Human Online
Authors: David Alan Grier

In spite of his French name, Crommelin was a British subject and an assistant astronomer at the Greenwich Observatory. He reported to George Airy's successor and oversaw the observatory's program of data reduction. The computing staff included ten full-time adult computers and twenty-six occasional workers, who reduced observations or prepared ephemerides when the research demanded it. They occupied a new facility behind the original observatory building which was equipped with calculating machines, slide rules, mathematical tables, and other computing aids.
4
This office structure, which combined the central computing room of William Stratford with the part-time workers of Nevil Maskelyne, could also be found in business offices of the time. Increasingly, office managers were forming a single pool of secretaries and stenographers to handle normal correspondence and were relying on outside workers for the extra demand of peak seasons. The United States Navy had also adopted this model for their computers, combining the calculating staffs of the Naval Observatory and the American
Nautical Almanac
into a single staff of eight, and relying on the contributions of twenty part-time computers at key times of the year, such as during the final preparation of the almanac.
5
With the Greenwich computing staff at his disposal, Crommelin could have been tempted to improve Pontécoulant's calculations simply by devoting all of his resources to the task. He could have divided the calculation into smaller steps and assigned all of his computing staff to the problem. Instead, he returned to the original analysis of the comet's motion and removed a key element that had been the basis for all cometary calculations, the elliptical orbit. Beginning with Edmund Halley's work in 1695, astronomers had assumed that the comet followed the idealized orbit of an ellipse and computed how the planets stretched and altered this path. As a calculation stepped the comet around the sun, it would eventually place the object so far from its original orbit that astronomers would have to recalculate the ellipse. This adjustment was a time-consuming task, but it was essential in order to maintain the accuracy of
the work. It “is a frequently troublesome process and does away with much of the advantage that there is in assuming that the comet is moving in an ellipse,” wrote Crommelin.
6
Rather than attempting to improve Ã
ngström's work or correcting the equations of Pontécoulant, Crommelin argued that he would get the best results by “discarding the elliptical hypothesis altogether and proceeding by mechanical quadratures.”
7
Mechanical quadratures is a method for solving differential equations, the basic mathematical expressions that model the motion of bodies in space. Differential equations describe relationships between the position of an object, its velocity, and the forces acting upon it. Newton solved a differential equation when he analyzed the way in which one body moves around another under the influence of gravity. He had used the techniques of calculus to solve this equation and discovered that one of the possible results was an ellipse. Mechanical quadratures, a technique now called “numerical integration,” was an alternative to Newton's calculus. It solves a differential equation solely by numerical methods, with no reference to the original ellipse or any other curve.
Crommelin, with the assistance of an observatory colleague, Phil Crowell (1879â1949), identified the basic differential equations that described the path of the comet and created a computing plan for the Greenwich Observatory computing staff. The computing plan had certain similarities to the plan that Clairaut had used in 1757. It located all the key objects in space and described the forces acting between them. At each step of the calculation, the computers advanced the comet, Saturn, Jupiter, and the other planets forward by a small distance. They did not worry about elliptical orbits but instead followed the direction of the forces. Once they had moved the objects, they had to recalculate all the forces. It was a slow and methodical process, one that required much grinding of Brunsvigas and other calculating machines. Without adding machines, only the most dedicated computers would attempt to use mechanical quadratures to compute the orbit of Halley's comet. Even though Crommelin and Crowell had the assistance of the Greenwich Observatory computing staff, they found that the work took longer than they had anticipated. “Owing to the pressure of time it has not been possible to do the whole of the work in duplication,”
8
they confessed. They were able only to confirm that there were no gross errors in the result, which suggested that the perihelion would occur on April 17.
When Halley's comet appeared as a faint speck in September 1909, the two astronomers returned to their calculations in order to get an exact date for the perihelion. Rather than recalculate the entire orbit using mechanical quadratures, they simply employed the traditional formula for an elliptical orbit. “This assumption is amply sufficient to give the date of
perihelion,” they wrote.
9
This calculation gave a date of April 20, and though this result was of some interest to astronomers, it proved to be of limited importance to the larger public in both Europe and the United States. Those outside of the scientific community looked for some larger meaning in the comet, something beyond the periodic cycling around the
sun of a material lump. The author Mark Twain saw it as the grand end of his era. “I came in with Halley's Comet in 1835,” he wrote. “It will be the greatest disappointment of my life if I don't go out with Halley's Comet.”
10
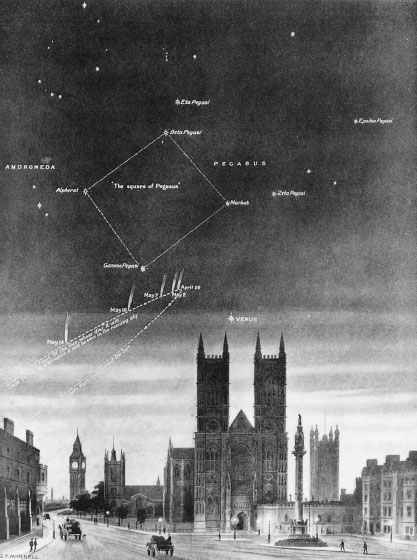
19. Path predicted for Halley's comet in 1910 as seen from London
“We had the sky up there, all speckled with stars,” Twain had written in his novel
The Adventures of Huckleberry Finn
, “and we used to lay on our backs and look up at them, and discuss about whether they was made or only just happened.” Twain's protagonist could not believe that anyone or anything could have created the stars because “it would have took too long to make so many,” but he conceded that they might have been laid, like the eggs of a frog. In the years that had passed since Twain wrote this novel, astronomers had developed some tools that could gather information on the constitution and origin of the comets. By dividing the reflected light of the comet into its individual colors, they were able to identify some of the substances to be found in its head and tail. Such information was of interest to an age that was anxious about the fact that the Earth would pass through the tail of the comet. A French astronomer was quoted in the
New York Times
as stating that the comet “would impregnate the atmosphere [of the Earth] and possibly snuff out all life on the planet.”
11
Of all the predictions, both good and ill, that circulated in the spring and winter of 1910 concerning the comet, only Twain's prediction of his own demise proved to be accurate. Twain died on April 20, 1910, just as the comet was passing through its perihelion and was starting on its outbound course.
As others had before him, Crommelin and his staff returned to their calculations after the comet passed and assessed the accuracy of their results. Taken as a group, the calculations of 1835 fell within sixteen days of the true date of perihelion. The single 1910 computation was within two days, sixteen hours, and forty-eight minutes of the correct value, an improvement by a factor of about five. Crommelin claimed that the method of mechanical quadratures, “if pressed to the extreme accuracy of which it is capable, will give results of higher degrees of accuracy than any previously published method of dealing with this comet.” He attempted to demonstrate the validity of this claim by making a more refined calculation of the comet's orbit. After expending much effort on the computation, he found that his revised work was no better than his original estimate. He did not withdraw his claims for mechanical quadratures but instead speculated that something other than mathematical accuracy was causing the discrepancy. He suggested that some additional force, an interstellar drag, was slowing the progress of Halley's comet.
12
After the comet had again been lost to view in the outer reaches of the solar system, just a few years after Crommelin had complained that no was one engaged in computing the orbit of Halley's comet, the English
mathematician Edmund Whittaker (1873â1957) wrote that “there has been a great awakening of interest in [computation]; and it is now included in the syllabus for the [British Civil Service] Examination.” The calculation of a comet's orbit might no longer pose an opportunity for new discoveries, but there were other fields that looked to scientific calculation as a way of providing detailed, precise answers. Whittaker observed that a knowledge of calculation was “required by workers in many different fieldsâastronomers, meteorologists, physicists, engineers, naval architects, actuaries, biometricians, and statisticians.”
13
In 1913, he was a professor at the University of Edinburgh, in Scotland, and was in the process of forming a laboratory devoted to the topic of computation. Most of his efforts were devoted to cataloging the methods of calculation and to creating new techniques that might be applied to broad classes of problems, just as the method of mechanical quadratures might be applied to problems other than those of comet orbits.
Whittaker had been trained as an astronomer and, for a time, had served as the Astronomer Royal of Ireland. Like other astronomers before him, Whittaker had been drawn to the mathematics of insurance and, according to his biographer, had “been influenced by his friendship with the great actuaries of the period.”
14
His laboratory borrowed from actuarial practice, a practice that demanded that computers follow calculating plans to the letter and that they record their intermediate values in ways that would allow others to verify their work. Whittaker gave detailed recommendations for every aspect of computing. He told computers to write numbers in pairs, for it is “found conducive toward accuracy and speed,” and argued that “every computation should be performed with ink in preference to pencil; this not only ensures a much more lasting record of the work but also prevents eyestrain and fatigue.” He felt that all computation should be done on specially prepared paper that was “divided by a faint ruling into 1/4 squares, each of which is capable of holding two digits.” His recommendations even included the desks that should furnish the computing room. He stated that the desks “used in the mathematical laboratory of the University of Edinburgh are 3â² 0â³ wide, 1â² 9â³ from front to back and 2â² 6½Ⳡhigh. They contain a locker, in which computing paper can be kept without being folded, and a cupboard for books, and are fitted with a strong adjustable book-rest.”
15
Beginning with his first writings on the subject, Whittaker was a strong advocate for direct numerical calculation. Many scientists still relied on graphical means for calculation. They would find a way of constructing some two-dimensional shape that had an area equal to the desired result. Once the shape was finished, the correct answer could be determined by measuring the area of the shape. Edmund Halley had used such a method when he first looked at the orbits of comets. Just as Halley had abandoned
this technique, Whittaker advised others to do the same. He wrote that at his Edinburgh laboratory “graphical methods have almost all been abandoned, as their inferiority has become evident, and at the present time the work of the Laboratory is almost exclusively arithmetical.”
16
The staff of the Edinburgh Mathematics Laboratory worked on a variety of problems, but they devoted most of their efforts to the construction of mathematical tables, to computing values of complicated mathematical functions that appeared in many different forms of scientific or engineering endeavor. Simple examples of mathematical tables include the familiar pages of logarithms, sines, and tangents and the probability functions of Karl Pearson and his Biometrics Laboratory. The Edinburgh computers tried their hands at such tables, but they were most interested in tabulating the Bessel function. The Bessel function was named for Friedrich Wilhelm Bessel (1784â1846), a German scientist who had been known as a mathematician and as the director of an astronomical observatory in Königsberg. “Many mathematicians, usually working in celestial mechanics, arrived independently at the Bessel function,” wrote the historian Morris Kline.
17
Bessel wrote the first systematic treatment of this function in 1824 as an outgrowth of his study of planetary motion. The task of tabulating this function was substantial, as it appeared in two different forms and behaved in a wide variety of ways. In the late nineteenth century, it emerged as one of the great problems for scientific computers. The orbit of Halley's comet was computed once every 75 years and then packed away for another generation. The Bessel function found applications in ever-broadening fields of science. It was useful to anyone studying problems of vibration, including vibrating drums, vibrating air, and vibrating electrical signals. It also could be applied in the study of heat and the diffraction of light.
18
As scientific computing moved from Greenwich to Edinburgh, from Halley's comet to the Bessel function, it slipped, at least slightly, from the grasp of astronomers and was picked up by scientists who were studying phenomena that were much closer to the earth.
