The Reenchantment of the World (9 page)

 

 

Although it is not possible, in this brief discussion, to elaborate in any
greater detail on Galileo's intellectual antecedents, some comments on
Tartaglia are in order because his works and style provide a major clue
to Galileo's methodology. New Science was the earliest attempt to apply
mathematics to projectiles, and it dealt extensively with the trajectories
of cannonballs. Tartaglia was first to break with the Aristotelian notion
of discontinuous trajectories, to state that the projectile path was
curvilinear, and to demonstrate that the maximum range of a projectile
occurred at a gun elevation of 45 degrees. Contradicting Aristotle, he
claimed that the air resisted motion, rather than assisting it. Between
the covers of a book on ballistics, then, Tartaglia advanced a theoretical
analysis of motion. This same combination occurred in a book he wrote in
1551 on the raising of sunken vessels, a topic of obvious interest to a
republic like Venice. To this study he appended his Italian translation of
Archimedes' essay "On Bodies in Water." Again, the text emerged not merely
as a technical treatise, but as the first open challenge to Aristotle's
law of falling bodies, for it used Archimedes' theory of buoyancy
and surrounding media to argue against Aristotle's rigid distinction
between up and down. Galileo was to follow in Tartaglia's footsteps,
arguing that there was no natural upward motion; using Archimedes to
overturn Aristotle; refining the mathematics of projectile motion; and
intimately connecting, as Tartaglia had done in all his work, technical
fieldwork with theoretical conclusions.

 

 

Galileo's involvement in technical problems was most intense during the
so-called Paduan period (1592-1610) when he was engaged in his studies of
motion. His own laboratory was like a workshop, where he manufactured
mathematical apparatus. Galileo tutored privately on mechanics and
engineering; did research on pumps, the regulation of rivers, and
fortress construction; and brought out his first printed work, on the
military compass, or "sector," as it was called. He also invented the
"thermobaroscope," and took a strong interest in the field of engineering
(now called materials science) which deals with the resistance of
materials. Although Galileo made a distinction in his own mind between
craft and theory, he broke with the prevailing view that saw them as
totally unrelated. He was not just a scientist who also happened to be
interested in technology, but rather used technology -- both in spirit and
method -- as the source of theory. His last work, the "Two New Sciences,"
opens with the following conversation between two imaginary interlocutors:

 

 

Salviati: The constant activity which you Venetians display in
your famous arsenal suggests to the studious mind a large field
for investigation, especially that part of the work which involves
mechanics; for in this department all types of instruments and
machines are constantly being constructed by many artisans, among
whom there must be some who, partly by inherited experience and
partly by their own observations, have become highly expert and
clever in explanation.

 

 

Sagredo: You are quite right. Indeed, I myself, being curious
by nature, frequently visit this place for the mere pleasure of
observing the work of those who, on account of their superiority
over other artisans, we call "first rank men." Conference with them
has often helped me in the investigation of certain effects including
not only those which are striking, but also those which are recondite
and almost incredible.16

 

 

The book not only contains a discussion of projectile motion, but also
includes a table of ranges for firing. Galileo makes much of the value
of his theory to gunners, but as it turns out, they did much more for
his science than he did for theirs.

 

 

How exactly did the technological tradition surface in Galileo's studies
of motion? He not only agreed with the literature of this tradition,
that construction is a mode of cognition, that manipulating nature
is a key to knowing it, but he also showed precisely how this type of
investigation should be carried out.

 

 

The analysis of projectile motion, of course, was derived from a
practical military problem, and was, at the same time, a crucial blow
to Aristotelian physics. Since Aristotle divided motion into two types,
forced and natural, he concluded that projectile motion (see Figure 9)
had to be discontinuous, that is, it had to consist of a forced motion
(throwing the object into the air) and a natural one (the descent
to earth):

 

 

 

 

 

When people first hear about this theory, they often ask how intelligent
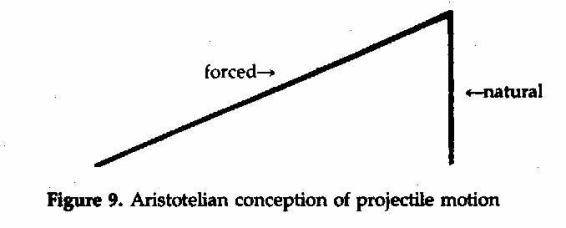
men and women could have believed it, since all one has to do is look
at a projectile to see that the above "curve" does not correspond
to reality. In fact, the acceptance of Aristotle's theory is a good
example of the gestalt principle of finding what you seek. Most readers
probably have not watched a projectile very closely, and certainly
few have plotted on a graph exactly where its apogee occurs and what
then takes place. Furthermore, from the point of view of the thrower,
a stone does seem to rise and then vertically drop. Finally, not until
the end of the sixteenth century were cannon fired at long range,
so such motion was not typically a part of the environment. As late
as 1561 graphs in some textbooks were superimposed over a cannon, with
the motion of the ball being shown as discontinuous (see Plate 1). In a
world of qualitative science, the Aristotelian picture is roughly "true"
in that it is one apparent aspect of projectile motion. Only with the
rise of standing armies and the military concentration on ballistics
was there any interest at all in a precise mathematical description of
cannonball flight, which in any case is never really parabolic (see below)
due to the effects of air resistance. We thus see how blurry, or complex,
a simple "fact" can be: it seems to be shaped by what is being asked.

 

 

In any event, closer and closer scrutiny of projectiles made it more
difficult to maintain the Aristotelian distinction between forced and
natural motion. Since it is virtually impossible to map the points on
a graph for an object actually thrown into the air, Galileo once again
abstracted the essentials of the situation and adapted them to laboratory
conditions. Projectile motion, he reasoned, is a free-fall situation with
a horizontal component. At the apogee of the curve, the object starts
its downward descent due to the force of gravity, but it still retains
some of the horizontal impetus originally imparted to it. The path
would thus be smooth, not discontinuous, as Aristotle had maintained;
and rather than abruptly falling to earth in a sheer vertical drop, the
object would describe a curve, a combination ("resultant") of the vertical
and horizontal components of motion. Galileo's experiments to ascertain
this curve mathematically involved rolling a ball down an inclined plane
that had a horizontal deflector at the bottom, and which was sitting on
the edge of a table. The ball was released from different points along
the plane, and thus in each trial struck the floor at a correspondingly
different point. This generated a mass of data -- really a collection of
curves-- which enabled Galileo, using his law of free-fall, to derive a
mathematical description of these curves as parabolic. In a nonresistant
medium, he finally concluded, the trajectory of a projectile would be
a perfect parabola. '

 

 

 

 

 

 

 

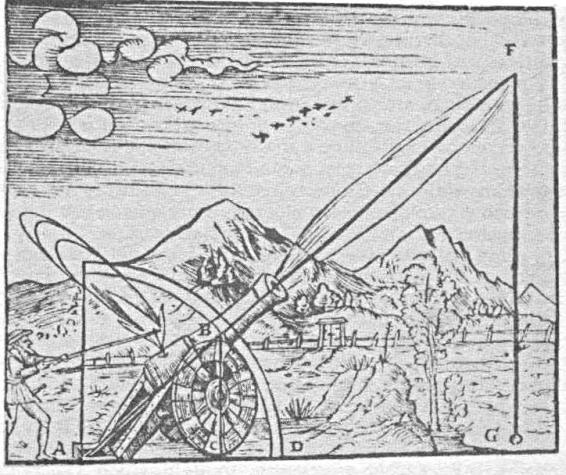
Plate 1. The Aristotelian theory of projectile motion, from Daniele
Santbech, "Problematum Astronomicorum" (1561). Courtesy Ann Ronan
Picture Library.

 

 

 

 

The significance of this was not merely the mathematical description of
a curve, but the challenge to Aristotelian physics. Not only did this
weaken the distinction between forced and natural motion; it also called
into question Aristotle's assertion that vacua could not exist (since
projectile motion was supposedly maintained by displacing air rushing
in to prevent a vacuum from forming), as well as the whole concept of
immanent purpose contained in the Aristotelian doctrines of natural
motion and natural place. Galileo's discovery of the independence of the
horizontal and vertical components of motion, which is another aspect of
the above investigation, led to his formulation of the composition and
resolution of forces -- what we now call vector mechanics. Here again,
measurement, rather than any sort of purpose, is seen to lie at the
heart of scientific explanation (if so it can be called). We see, then,
that a military problem, which had been investigated by an engineer
like Tartaglia, was converted into a controlled laboratory experiment
to produce a mathematical expression, and then used to smash several
fundamental tenets of the Aristotelian world view. Galileo's studies
of ballistics not only refuted Aristotelian concepts; they were also
beginning to delineate a new method for exploring reality.

 

 

All of Galileo's investigations served as vivid demonstrations of the
relationship between theory and experiment which was slowly forming in
the minds of a few European thinkers. They also vindicated the unproven
assumption made by the technological literature of the sixteenth century:
there can be a fundamental link between cognition and manipulation,
between scientific explanation and mastery of the environment. The
economic history sketched in the early pages of this chapter is thus
much more than an interesting backdrop to these developments in the
seemingly abstract realm of scientific thought. Cognition, reality,
and the whole Western scientific method are integrally related to the
rise of capitalism in early modern Europe.

 

 

We have talked in terms of a gestalt principle, of facts being plastic,
"created" by theoretical constructs that are in turn linked to
a socioeconomic context; and of the Scientific Revolution and its
methodology as being part of a larger historical process. We are then
brought face to face with an unsettling question: Is reality nothing more
than a cultural artifact? Are Galileo's discoveries not the hard data of
science, but simply the products of a world view that is a more or less
localized phenomenon? If, as the foregoing analysis suggests, the answer
is yes, we are cast adrift on a sea of radical relativism. There is then
no Truth, but merely your truth, my truth, the truth of this time or that
place. This is the implication of what is commonly called the sociology of
knowledge. The distinction between knowledge and opinion, between science
and ideology, crumbles, and what is right becomes a matter of majority
rule, or "mob psychology."17 Modern science, astrology, witchcraft,
Aristotelianism, Marxism, whatever -- all become equally true in the
absence of objective knowledge and the concept of a fixed, underlying
reality. Is there no way to protect ourselves from such a conclusion?

 

 

My answer is that radical relativism arises out of the peculiar attitude
that modern science has adopted toward participating consciousness, which
I discussed very briefly in the Introduction. It will be necessary, in the
first place, then, to analyze the nature of participating consciousness
in some detail. To do so, we must pursue the sociology of knowledge
into a neglected chapter in the story of the Scientific Revolution:
the world of the occult.

 

 

 

 

 

 

 

 

 

 

3
The Disenchantment
of the World (1)

 

 

What appears a wonder is not a wonder.
-- Simon Stevin

 

 

 

 

The phrase is Weber's: 'die Entzauberung der Welt.' Schiller, a century
earlier, had an equally telling expression for it: 'die Entgötterung der
Natur,' the 'disgodding' of nature. The history of the West, according
to both the sociologist and the poet, is the progressive removal of mind,
or spirit, from phenomenal appearances.