Pythagorus (25 page)

By the mid-first century B.C., a cultlike group flourished in Rome under the leadership of Nigidius Figulus, a âPythagorean and magus' in whose Pythagoreanism the line between science and magic grew fuzzy to the point of extinction. Pythagoreanism âfor Nigidius and his friends meant primarily a belief in magic', wrote the historian Elizabeth Rawson.
8
Nigidius' reputation for having second sight and occult powers qualified him to work up a birth horoscope of the later-to-be-emperor Augustus, which correctly foretold a brilliant future. Romans of that era did not consider such a scholar out of the mainstream or on the lunatic fringe. Cicero wrote in the introduction to his own translation of Plato's
Timaeus
that Nigidius âarose to revive the teachings of the Pythagoreans which, after having flourished for several centuries in Italy and Sicily, had in some way been extinguished', and that he was âa particularly acute investigator of those matters which nature has made obscure'.
9
Nigidius was an educated, prolific author of books on the planets, the zodiac, grammar, natural philosophy, dreams, and theology, with an extensive knowledge of religions and cults from much of the known world.
Romans often invoked Pythagoras' name to represent wisdom and integrity. The scholar and satirist Marcus Terentius Varro, considered by many the most learned Roman of the first century B.C., began his book
Hebdomades
with Pythagorean-sounding praise of the number 7 and a quotation about astronomy from Nigidius. When Varro died he was buried, according to Pliny, in the âPythagorean mode', in a clay coffin with myrtle, olive, and black poplar leaves.
10
Cicero, for his part, attempted to undermine the credibility of one âVatinius', a supporter of Julius Caesar, by righteously accusing him of impiety: for he âcalls himself a Pythagorean and, with the name of that most thoroughly learned man, tries to shield his monstrous, barbarous behaviour.'
11
Cicero seems never to have joined a Pythagorean cult, but he made a pilgrimage to Metapontum to visit the house where tradition said Pythagoras died.
Pythagoras made appearances in many of Cicero's works. In a scene from
The Republic,
set at Scipio Africanus' country estate, Africanus and his nephew Quintus Tubero, the first of several expected visitors to arrive, recline on couches in the Roman fashion, awaiting another guest, Panaetius, who investigates problems of astronomy âwith the greatest enthusiasm'. In anticipation of his arrival, Scipio mentions a matter that has come up in the Senate about a âsecond sun',
[5]
then remarks,
SCIPIO AFRICANUS:
I prefer to be guided by Socrates, who wisely declined all such speculation, saying that the investigation of nature was far above human reason, or would contribute little to the enjoyment of human life.
TUBERO:
I know not, Africanus, why it should be reported that Socrates declined all such investigations and confined himself to those which touched on life and manners. For what author is fuller of his praise than Plato, in whose books, in numerous places, Socrates is represented as discoursing not only on morals, on virtue, and on politics, but, as Pythagoras did, on numbers, geometry, and harmony.
SCIPIO:
It is as you say; but, I believe, Tubero, you have heard that Plato, after the death of Socrates, in order to acquire information, visited first Egypt, and then Italy and Sicily, that he might make himself acquainted with the theories of Pythagoras; and he appears to have associated very much with Archytas of Tarentum and with Timaeus the Locrian,[
[6]
] and to have studied the commentaries of Philolaus. Observing at that time that the name of Pythagoras flourished in those parts, he devoted himself to Pythagorean studies and associates. As he had become especially charmed with Socrates, to whom he attributed everything, he managed to mingle a certain subtlety peculiar to Socrates with the mystery of Pythagoras and his profound knowledge of many of the arts.
12
Tubero thinks of Pythagoras in connection with arithmetic, geometry, and harmony. Scipio associates him with mysticism and profound, âvaried lore'. Later in the same conversation, they invoke his authority on the natural foundation of laws protecting life:
No ordinary men, but the ablest and best educated, such as Pythagoras and Empedocles, have declared that there should be only one rule of law for all living animals, and they would condemn to eternal punishment those by whom any animal was injured.
13
Cicero even weighed in on the bean issue: Pythagoreans avoided them because they cause âconsiderable flatulence and thus are inimical to those who seek peace of mind'.
14
It was in Cicero's âDream of Scipio' that he sounded most Pythagorean â and also much like Plato. The âDream' concluded Cicero's
De republica
, and in a graceful parallel, he modelled it on the âMyth of Er' that ended Plato's
Republic
. Cicero's âDream' takes him to a region accessible only to those who through music, learning, genius, and devotion to divine studies have achieved permanent reunion with the highest level of existence. His ears are filled with a sound âstrong and sweet', and he asks Scipio what it is. Scipio replies,
That is caused by the impulse and motion of the orbs themselves, which are separated by precise but unequal intervals, set in exact proportion, high sounds mingling with low, producing a variety of harmonies in equal degree; nor can such rapid movement be excited without noise, for nature has ordained that sound shall be ordered from the extremities of low at one end to high at the other. Hence that star of the heaven whose course is the highest, and whose revolution is very rapid, moves with a sharp and quick sound, whilst the moon, being the lowest, moves with a very low, heavy sound; and the earth, the ninth, remains immovable; always occupying the same seat, fixed in the middle of the universe.
15
Because Venus and Mercury âare in unison', there are only seven sounds â matching the number of strings on the seven-stringed lyre â âseven distinct sounds with equal intervals'. By imitating this harmony with strings and voices, âlearned men have endeavoured to win their way back to this place, whilst others, endowed with pre-eminent ability, have, during their whole lives, cultivated these divine studies.'
16
Cicero's metaphor to explain why most humans never hear the celestial music was that their ears are deafened to the sound, âlike those people on the Nile at the place called Catadupa, who, living where the waters fall from very high mountains, have by its roar lost the sense of hearing.'
17
He gave no indication that he knew Pythagoreans had thought the Earth was not the centre of the cosmos. In fact, nowhere in the surviving ancient literature is there a hint of anyone bringing the concept of an audible âmusic of the spheres' together with the cosmology that included the central fire and the counter-earth, even though the musical ratios had probably played a role in the development of the Pythagorean ten-body model of the cosmos.
In a different realm of scholarship, one extremely successful younger Roman contemporary of Cicero, the architect Marcus Vitruvius Pollio, authored an overview of architecture of his era,
De architectura
or
Ten Books on Architecture
. He recommended Pythagorean ratios and extrapolations on them for the dimensions of rooms, not using any shapes for temples other than one whose length was twice its width (ratio 2:1), or circular. Greek forums were square, but Vitruvius' had a width 2/3 its length, because an audience for gladiatorial combat was better accommodated in that space. For houses, âthe length and breadth of courts [atria] are regulated in three ways', two of which employed Pythagorean ratios: âThe second, when it is divided into three parts, two are given to the width'. The third: âA square being described whose side is equal to the width, a diagonal line is drawn therein, the length of which is to be equal to the length of the atrium'.
18
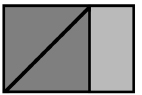
This design was based on Socrates' lesson in Plato's
Meno
. âBy numbers this cannot be done', wrote Vitruvius. Socrates had used no numbers. The length of that diagonal was incommensurable; so was the length of one side of Vitruvius' room. He frequently mentioned Pythagoras and the Pythagoreans. The Pythagorean theorem was a shortcut in designing staircases, and he unhesitatingly attributed it to Pythagoras.
Vitruvius' books had illustrations, but copies that reached the Renaissance did not. The drawing below, by Cesare Cesariano, is a Renaissance (1521) realisation of Vitruvius, who was not easy to interpret. According to the architect Leon Battista Alberti, âGreeks thought he was writing in Latin; Latins thought he was writing in Greek.' Nevertheless, this drawing probably faithfully represents his instructions:
This proposition is serviceable on many occasions, particularly in measuring [and] setting out the staircases of buildings so that each step has its proper height. If the height from the pavement to the floor be divided into three parts, five of them will be the exact length of the inclined line which regulates the blocks of which the steps are formed. Four parts, each equal to one of the three into which the height from the pavement to the floor was divided, are set off from the perpendicular for the position of the first or lower step. Thus the arrangement and ease of the flight of stairs will be obtained, as the figure shows.
19
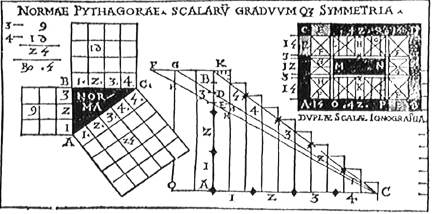
Drawing by Cesare Cesariano that represents a Renaissance realisation of Vitruvius' works
Vitruvius' book referred to an unusual application of musical fourths, fifths, and octaves used in an amplification system in Greek theatres. A Roman theatre, he pointed out, being made of wood, had good acoustics, but in a Greek theatre, made of stone, the voices of the actors needed amplification:
So [the Greeks placed vessels] in certain recesses under the seats of theatres, fixed and arranged with a due regard to the laws of harmony and physics, their tones being fourths, fifths, and octaves; so that when the voice of the actor is in unison with the pitch of these instruments, its power is increased and mellowed by impinging thereon.
20
This was by way of demonstrating that an architect must be the master of many subjects â not so difficult as it might seem, thought Vitruvius, for a very Pythagorean reason:
For the whole circle of learning consists in one harmonious system. . . . The astronomer and musician delight in similar proportions, for the positions of the stars answer to a fourth and fifth in harmony. The same analogy holds in other branches of Greek geometry which the Greeks call λóγοÏ ÏÏικòÏ: indeed, throughout the whole range of art, there are many incidents common to all.
ÏÏικòÏ: indeed, throughout the whole range of art, there are many incidents common to all.
