Phantoms in the Brain: Probing the Mysteries of the Human Mind (34 page)
Read Phantoms in the Brain: Probing the Mysteries of the Human Mind Online
Authors: V. S. Ramachandran,Sandra Blakeslee
Tags: #Medical, #Neurology, #Neuroscience

Nor do we have to invoke the extreme pathological example of savants to make this point, for there is an element of this syndrome in every talented person or indeed in every genius. "Genius," contrary to popular misconception, is not synonymous with superhuman intelligence. Most of the geniuses whom I have had the privilege of knowing are more like idiot savants than they would care to admit—extraordinarily talented in a few domains but quite ordinary in other respects.
Consider the oft−told story of the Indian mathematical genius Ra−manujan, who at the turn of the century worked as a clerk in the Madras seaport, a few miles from where I was born. He had matriculated to the early part of high school, where he performed badly in all his subjects, and he had no formal education in advanced mathematics. Yet he was astonishingly gifted in math and was obsessed by it. So poor that he couldn't afford paper, he would use discarded envelopes to scribble his
135
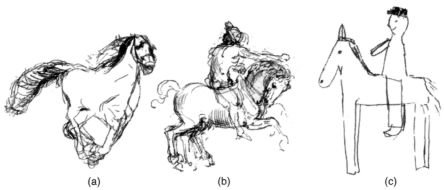
Figure 9.2
(a) A drawing of a horse made by Nadia, the autistic savant, when she was five years old. (b) A
horse drawn by Leonardo da Vinci, (c) A drawing of a horse by a normal eight−year−old. Notice that Nadia's
drawing is vastly superior to that of the normal eight−year−old and almost as good as (or perhaps better
than!) da Vinci's horse,
(a) and (c) reprinted from
Nadia,
by Loraa Selfe, with permission from Academic Press (New York).
mathematical equations, discovering several new theorems before the age of twenty−two. Since he was not acquainted with any number theorists in India, he decided to communicate his discoveries to several mathematicians in other parts of the world, including Cambridge, England. One of the world's top number theorists of that time, G.H. Hardy, received his scribbles and immediately thought Ramanujan was a crackpot.
Having glanced at them, he went out to play tennis. As the game wore on, Ramanujan's equations kept haunting him. He kept seeing the numbers in his mind. "I had never seen anything in the least like them before," Hardy later wrote. "They must be true because no one would have had the imagination to invent them." So he promptly went back and checked the validity of the elaborate equations on backs of envelopes, saw that most of them were correct and immediately sent a note to his colleague J.E. Littlewood, who also went over the manuscripts. Both luminaries quickly realized that Ramanujan was probably a genius of the highest caliber. They invited him to Cambridge, where he worked for many years, eventually surpassing them in the originality and importance of his contributions.
I mention this story because if you were to go out to dinner with Ramanujan you wouldn't think there was anything unusual about him. He was just like any other person except for the fact that his mathematical skills were way off scale—almost supernatural, some have said. Again, if mathematical ability is simply a function of general intelligence, a result of the brain's getting bigger and better overall, then more intelligent people should be better at math, and vice versa. But if you met Ramanujan, you'd know that that just isn't true.
What is the solution? Ramanujan's own "explanation"—that the fully formed equations were whispered to him in dreams by the presiding village deity, Goddess Namagiri—doesn't really help us very much. But I can think of two other possibilities.
The first, more parsimonious, view is that general intelligence is really a number of different mental traits—with both the genes and the traits themselves influencing each other's expression. Since genes combine randomly in the population, every now and then you will get a fortuitous combination of traits—such as vivid visual imagery combined with excellent numerical skills—and such shuffling can throw up all sorts of unexpected interactions. Thus is born that extraordinary flowering of talent we call genius—the gifts of an Albert Einstein who could "visualize" his equations or a Mozart who saw, and did not merely hear, his musical compositions unfold in his mind's eye. Such genius is rare only because the lucky genetic combinations are rare.
136
But there's a problem with this argument. If genius results from serendipitous genetic combinations, how does one explain the talents of Nadia and Tom, whose general intelligence is abysmal? (Indeed, an autistic savant's social skills may be less than those of a Bonobo ape.) It's difficult, moreover, to see why such unique talent should actually be
more
common among savants than it is among the general population, who, if anything, have a larger number of healthy traits to shuffle around in each generation. (As many as 10 percent of autistic children have perfect pitch, compared with only 1 or 2 percent of the general population.) Furthermore, the traits in that individual would have to "interlock" precisely and interact in such a way that the outcome is something elegant rather than nonsensical, a scenario that is as unlikely as a confederacy of dunces producing a work of artistic or scientific genius.
This brings me to the second explanation for the savant syndrome in particular and for genius in general. How can someone who can't tie shoelaces or carry on a normal conversation calculate prime numbers? The answer might lie in a region of the left hemisphere called the angular gyrus, which, when damaged, leaves some people (like Bill, the Air Force pilot in Chapter 1 who couldn't subtract) with an inability to do simple calculations, such as subtract 7 from 100. This does not mean that the left angular gyrus is the brain's math module, but it's fair to say that this
structure is doing something crucial for mathematical computation and is not essential for language, working memory or vision. But you do seem to need the left angular gyrus for math.
Consider the possibility that savants suffer early brain damage before or shortly after birth. Is it possible that their brains undergo some form of remapping as seen in phantom limb patients? Does the prenatal or neonatal injury lead to unusual rewiring? In savants, one part of the brain may for some obscure reason receive a greater than average input or some other equivalent impetus to become denser and larger—a huge angular gyrus, for example. What would be the consequence for mathematical ability? Would this produce a child who can generate eight−digit prime numbers? In truth, we know so little about how neurons perform such abstract operations that it's difficult to predict what the effect of such a change might be. An angular gyrus doubled in size could lead not to a mere doubling of mathematical ability but to a logarithmic or hundredfold increase. You can imagine an explosion of talent resulting from this simple but "anomalous" increase in brain volume. The same argument might hold for drawing, music, language, indeed any human trait.12
This argument is zany and unashamedly speculative, but at least it's testable. A math savant should have a large or hypertrophied left angular gyrus, whereas an artistic savant may have a hypertrophied right angular gyrus. Such experiments have not been done, to my knowledge, although we do know that damage to the
right
parietal cortex, where the angular gyrus is located, can profoundly disrupt artistic skills (just as damage to the left disrupts calculation).
A similar argument can be put forth to explain the occasional emergence of genius or extraordinary talent in the normal population, or to answer the especially vexing question of how such abilities cropped up in evolution in the first place. Maybe when the brain reaches a critical mass, new and unforeseen traits, properties that were not specifically chosen by natural selection, emerge. Maybe the brain had to become big for some other more obviously adaptive reason—throwing spears, talking or navigation—and the simplest way to achieve this was to increase one or two growth−related hormones or morphogens (genes that alter size and shape in developing organisms). But since such a hormone− or morphogen−based growth spurt cannot selectively increase the size of some parts while sparing others, the bonus might be an altogether bigger brain, including an enormous angular gyrus and the accompanying tenfold or hundredfold enhancement in mathematical ability. Notice that this argument is very different from the widely held belief that you de−
velop some very "general" ability that is then deployed for a specialized skill.
137
Taking this speculation even further, is it possible that humans find such esoteric talents—be it music, poetry, drawing or math—to be sexually attractive mainly because they serve as an externally visible signature of a giant brain? Just as the peacock's large, iridescent tail or the size of a majestic bull elephant's tusks constitutes
"truth in advertising" for the animal's health, so the human ability to croon a tune or pen a sonnet might be a marker for a superior brain. ("Truth in advertising" may play an important role in mate selection. Indeed, Richard Dawkins has suggested, half seriously, that the size and strength of a human male's erection may be markers for general health.)
This line of reasoning raises some fascinating possibilities. For instance, you could inject hormones or morphogens into a fetal human brain or infant to try to increase brain size artificially. Would this result in a race of geniuses with superhuman talents? Needless to say, the experiment would be unethical to do in humans, but an evil genius might be tempted to try it on the great apes. Is so, would you see a sudden efflorescence of extraordinary mental talents in these apes? Could you accelerate the pace of simian evolution through a combination of genetic engineering, hormonal intervention and artificial selection?
My basic argument about savants—that some specialized brain regions may have become enlarged at the expense of others—may or may not turn out to be correct. But even if it's valid, bear in mind that no savant is going to be a Picasso or an Einstein. To be a true genius, you need other abilities, not just isolated islands of talent. Most savants are not truly creative. If you look at a drawing by Nadia, you do see creative artistic ability,13 but among mathematical and musical savants, there are no such examples. What seems to be missing is an ineffable quality called creativity, which brings us face to face with the very essence of what it is to be human. There are those who assert that creativity is simply the ability to randomly link seemingly unrelated ideas, but surely that is not enough. The proverbial monkey with a typewriter will eventually produce a Shakespeare play, but it would need a billion lifetimes before it could generate a single intelligible sentence—let alone a sonnet or a play.
Not long ago when I told a colleague about my interest in creativity, he repeated the well−worn argument that we simply toss ideas around in our heads, producing random combinations until we hit on aesthetically pleasing ones. So I challenged him to "toss around" some words and ideas by coming up with a single evocative metaphor for "taking things
to ridiculous extremes" or "overdoing things." He scratched his head and after half an hour confessed that he couldn't think of anything all that original (despite his very high verbal IQ, I might add). I pointed out to him that Shakespeare had crammed five such metaphors in a single sentence: To gild refined gold, to paint the lily, to throw a perfume on the violet, to smooth the ice, or add another hue unto the rainbow ... is wasteful and ridiculous excess.
It sounds so simple. But how come Shakespeare thought of it and nobody else? Each of us has the same words at our command. There's nothing complicated or esoteric about the idea that's being conveyed. In fact, it's crystal clear once it is explained and has that universal "why didn't I think ofthat?" quality that characterizes the most beautiful and creative insights. Yet you and I would never come up with an equally elegant set of metaphors by simply dredging up and randomly shuffling words in our minds. What's missing is the creative spark of genius, a trait that remains as mysterious to us now as it did to Wallace. No wonder he felt impelled to invoke divine intervention.
The W oman Who Died Laughing
God is a comedian performing before an audience that is afraid to laugh.
138
—
Friedrich Nietzsche
God is a hacker.
—
Francis Crick
On the morning of his mother's funeral in 1931, Willy Anderson—a twenty−five−year−old plumber from London—donned a new black suit, clean white shirt and nice shoes borrowed from his brother. He had loved his mother very much and his grief was palpable. The family gathered amid tearful hugs and sat silently through an hour−long funeral service in a church that was much too hot and stuffy. Willy was relieved finally to get outdoors into the chilly open air of the cemetery and bow his head with the rest of the family and friends. But just as the gravediggers began lowering his mother's roped casket into the earth, Willy began to laugh. It started as a muffled snorting sound that evolved into a prolonged giggle. Willy bowed his head farther down, dug his chin into his shirt collar and drew his right hand up to his mouth, trying to stifle the unbidden mirth. It was no use. Against his will and to his profound embarrassment, he began to laugh out loud, the sounds exploding rhyth−
