Life's Ratchet: How Molecular Machines Extract Order from Chaos (19 page)
Read Life's Ratchet: How Molecular Machines Extract Order from Chaos Online
Authors: Peter M. Hoffmann

Another important aspect of snow crystal growth is
mass transport
—how new water molecules can reach the growing crystal and become part of it. This is mostly a question of diffusion, the random motion of
water molecules in the vapor of clouds. A crystal can only grow as fast as water molecules can reach it. The crystal’s growth is
diffusion limited
. Physicists call such growth diffusion
-
limited aggregation. Again, parts of the crystal that stick out are easiest to reach by random diffusion (think of lightning rods, which are easiest to reach by the random motion of a lightning bolt), which adds to instability.
How complicated a simple snow flake is! The example of the snowflake illustrates how the interplay of energy, entropy, and various mechanisms (diffusion, heat transfer), together with an underlying geometry, can create complexity and variety, but also a certain robustness of structure. Snowflakes are not alive, but they serve to illustrate that complex structure formation, as encountered in living things, can arise from dynamic physical laws. Moreover, snowflakes are nonliving examples of structures that exhibit both repetition (all snowflakes are six-sided, spiky, and branchy) and nearly unlimited variety within this basic pattern.
Nanotechnologists use similar principles to create ever-more-complicated nanostructures. The ultimate goal is to design circuits or nanoscale machines that grow themselves, molecule by molecule. The simplest case of self-assembly occurs when spherical particles are deposited on a surface. If they have some attractive forces between them and some way to move around until they find a nice place to settle down (typically where they have one or more neighbors), they inevitably form close-packed layers. A close-packed layer looks like stacked oranges: Place (equal-size) oranges next to each other, so they all touch, and you end up with a close-packed, hexagonal layer. This is the most common structure found in nature (a honeycomb is a good example), because it maximizes contact between neighbors and wastes a minimum amount of space.
But hexagonal close-packing gets boring very quickly. If we want to make more complicated structures, we have to start with more complicated building blocks and with more innovative ways of putting these building blocks together. There are various ways to cajole molecular building blocks into forming complex structures. Use oddly shaped molecules that only attach to other molecules at certain attachment points (chemists call this directional bonding), or use nonequilibrium conditions that somehow bias the structure to form in a certain way (e.g., apply electrical fields, pressure, or a liquid flow), or let entropy give you a helping hand.
Mayonnaise is not good for you, but it sure tastes good on a sandwich. While it contains water and oil, it is remarkably stable and uniform, even though we know water and oil do not mix. If you’ve ever made mayonnaise (I tried once, but it wasn’t edible), you know the secret is that mayonnaise is made with egg yolks. But why would eggs stabilize an otherwise unstable mixture of oil and water?
Egg yolks are mostly water, with some proteins and a sizable amount of fat. The fat part consists of fatty acids (oils), lipids, and other compounds. Lipid molecules have two important parts: a salt
head
(which is water-soluble, or hydrophilic) and a fatty acid
tail
(which is water-insoluble, or hydrophobic). The molecules are a kind of conjoined twin, with each part having different affinities. In a mixture of oil and water, lipids can satisfy each part of their split personality. The hydrophilic head can stick out into water, while the hydrophobic tail can immerse itself in the oil. Lipids are just one example of such
amphiphilic
molecules (i.e., they are soluble in water
and
oil). Other examples are detergents, soaps, and other surfactants. Because of their dual solubility, these materials make oily, greasy stuff water-soluble and enable us to wash our dirty dishes.
This sounds good, but there is a problem: Oil and water molecules are always pushed around by the molecular storm. The unfortunate lipid or detergent molecule cannot enjoy its divided loyalties for long, before the oil and water mix again. How can these molecules get around this problem? They find strength in numbers. When they come together in sufficient numbers, they can form stable structures—
micelles
—spherical protective shields that completely engulf droplets of oil and permanently separate the oil from the surrounding water. Micelles are made of many, many lipid molecules. These structures allow the lipids to satisfy their conflicted hydrophilic-hydrophobic affinities while keeping the adversaries, oil and water, apart. The reduction of free energy associated with this arrangement is so large that the thermal motion of the molecular storm is too weak to break the micelles. The emulsion of oil and water has become stable. And that is why mayonnaise does not separate.
Micelles can also form in the absence of oil. If we place a single amphiphilic molecule into water, it would have no choice but to expose its
hydrophobic tail to the water. However, if there are enough molecules, they can form micelles, namely, spheres with an outer layer of hydrophilic heads and an inner core of hydrophobic tails. With the hydrophobic tails safely tucked away, this arrangement reduces free energy considerably. The formation of such micelles is a textbook example of cooperative dynamics. Cooperative dynamics, or cooperativity, plays a central role in structure formation in living systems. In a nutshell, cooperativity means that a structure cannot form unless a certain number of molecules “cooperate” to form the structure. In micelles, this means that a micelle requires a minimum number of lipid molecules to form. You need a certain number of molecules to form a complete sphere with a rather close-packed core of hydrophilic tails. Too few molecules, and you cannot form a closed sphere. Too many, and the sphere becomes too large, leaving a void in the center, which costs energy. Thus there is an optimal number of lipids in each micelle.
Imagine how a spherical micelle could form gradually. First two amphiphilic molecules meet and point their hydrophobic tails at each other. Then, they are joined by a third and so on, until the molecules form a closed sphere. But this is not at all what happens. Measurements show that below a minimum concentration of molecules in solution, no micelles form. The molecules remain lonely hearts. However, as more lipids are added, suddenly complete micelles start to form. Why is that? Micelles do not form gradually. Rather, at the critical micelle concentration, the probability that the correct number of molecules will spontaneously meet becomes large enough to allow the immediate formation of complete, stable micelles, without any partially assembled micelles along the way. Micelle formation is an all-or-nothing proposition. Either enough lipid molecules meet at the same place and cooperatively form a micelle, or no micelles form at all. It is like a proper soccer match: Either you (initially) have twenty-two players on the soccer field, or you don’t have a game.
One result of this all-or-nothing dynamic is that there is a very sharp change in the properties of a lipid solution when it reaches critical concentration. One such property is the
osmotic pressure
, that is, the pressure exerted on a wall due to the random motion of dissolved molecules (remember, pressure is just the sum total of all impacts of randomly moving molecules with a wall). In his famous papers on Brownian motion, Einstein showed that the osmotic pressure does not depend on the size of the
molecules or particles in solution, but depends only on their concentration and temperature. Thus, when micelles suddenly form from a large number of single molecules, the total number of particles in solution is greatly reduced. Where we originally had many lonely lipids, we now suddenly have just a few micelles—each counting as just one particle. This reduction in the number of free particles in solution leads to a sharp reduction in osmotic pressure. Such sharp, all-or-nothing transitions are a signature of molecular cooperativity.
Cooperativity, the observation that some structures form only when a minimum number of molecules cooperate, is ubiquitous throughout biology. Molecules do not cooperate in the sense that people cooperate. They are, after all, molecules. Molecules are pushed around randomly by the molecular storm. For example, if lipids can form a micelle, they will reduce their free energy enormously. If the concentration is too low, you can never get enough molecules together at the same location. But at some critical concentration, when you have enough molecules around, they find each other in sufficient numbers, and micelles form spontaneously.
Lipids can also form more complicated cooperative structures in water: double-walled spheres, called vesicles. Inside the double wall, the hydrophobic tails are safely tucked away from the surrounding water, while the two surfaces, one on the outside and one on the inside of the sphere, face water molecules (
Figure 4.1
). A vesicle separates one volume of water from another. If we place different chemicals inside the vesicle, we create an isolated, nanosize reaction chamber. All kinds of interesting things could go on inside this tiny chamber—life, for example. Indeed, the walls surrounding living cells are made of lipids; a living cell is in some sense a giant self-assembled vesicle with a lot of really complicated chemistry going on inside.
At the nanoscale, the molecular storm reigns supreme. Yet, the random thermal forces of the storm paradoxically lead to the creation of ordered structures. As a matter of fact, without it, nothing would be happening at all! Self-assembly requires that the pieces that make the structure are shuffled around until they find a comfortable resting place where they minimize
their free energy. As we have seen in
Chapter 3
, structures form sometimes to maximize random motion, that is, to maximize entropy.
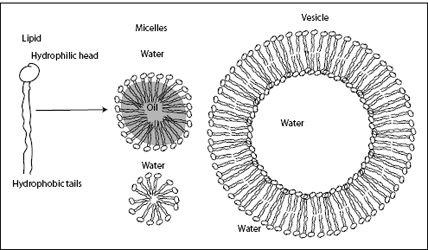
FIGURE 4.1.
Lipids are amphiphilic molecules with a hydrophilic head and two hydrophobic tails. In a water and oil mixture, lipids can surround small oil droplets and form micelles. Lipids can also form micelles in the absence of oil, but only if a specific number of lipids comes together to form a spherical shell. This is an example of cooperativity. Usually, however, lipids prefer to form vesicles, which are structures made of a double wall of lipids, separating one volume of water from another. Vesicles form the basis of cell membranes and are used inside cells to transport chemicals around.
Cells are incredibly crowded places, stuffed full of large proteins, DNA and RNA molecules, sugars, lipids, ions, and innumerable water molecules. It has been estimated that the average space between proteins in living cells is less than ten nanometers. Since proteins are between ten and one hundred nanometers in size, this is equivalent to a crowded parking lot with just a foot or less between each car. When things are this tight, it becomes tricky to maneuver past each other. In addition to this crowding, every space between proteins is filled with water, ions, sugars, and other assorted small molecules. You now have an idea of what a crowded mess a cell is. This crowding has consequences, many of which are not well understood, because when we do experiments on proteins, the steps are usually conducted outside the cell in a test tube, where proteins have plenty of space.
One surprising consequence of crowding is that the small guys (water, ions) rule over the big guys (proteins, DNA, lipid vesicles). Imagine two large protein molecules approaching each other in a sea of smaller molecules. The small molecules, because of their finite size, can only approach the big molecules so far. If they get any closer, they’d have to press into the large molecule. Thus, every large molecule is surrounded by an exclusion zone (also called a depletion zone) that cannot be penetrated by small molecules and thus leads to a reduction in total space available to the small molecules. It is a kind of molecular personal space: Imagine our large molecules as adults standing around a room, each with their own personal space, and the small molecules as kids running around. It is difficult for the children to run around, as they have to avoid entering the adults’ personal spaces. Suddenly, the adults pair off, and couples start to embrace. What previously were two personal spaces, one for each person, becomes one merged personal space per couple. Suddenly, more room is available for the kids to run around, and they promptly take advantage of their newfound freedom (
Figure 4.2
).
