I Am a Strange Loop (65 page)

Ultimately, the “I” is a hallucination, and yet, paradoxically, it is the most precious thing we own. As Dan Dennett points out in
Consciousness Explained,
an “I” is a little like a bill of paper money — it
feels
as if it is worth a great deal, but ultimately, it is just a social convention, a kind of illusion that we all tacitly agree on without ever having been asked, and which, despite being illusory, supports our entire economy. And yet the bill is just a piece of paper with no intrinsic worth at all.
Trains Who Roll
In Chapters 15 through 18, I argued that each of us is spread out and that, despite our usual intuitions, each of us is housed at least partially in different brains that may be scattered far and wide across this planet. This viewpoint amounts to the idea that one
can
be in two places at once, despite our initial knee-jerk rejection of such a crazy-sounding thought. If being in two or more places at once seems to make no sense, think about reversing the roles of space and time. That is, consider that you have no trouble imagining that you will exist tomorrow and also the next day. Which one of those future people will
really
be you? How can two
different
you’s exist, both claiming your name? “Ah,” you reply, “but I will shortly be getting there, like a train pulling through different stations.” But that just begs the question. Why is it the
same
train, if in the meantime it has dropped some passengers off and picked others up, perhaps changed a car or two, maybe even its locomotive? It is simply
called
“Train 641”, and
that’s
why it is “the same train”. It’s a linguistic convention, and a very good one, too. It is a very natural convention in the classical world in which we exist.
If Train 641, heading east from Milano, always were to split up in Verona into two pieces, one that headed north to Bolzano and one that continued eastwards to Venice, then we would probably not call either half “Train 641” any longer, but would give them separate numbers. But we could also call them “Train 641a” and “Train 641b”, or even just leave them both as “Train 641”. It might happen, after all, that upon reaching Bolzano, the northern half always veers suddenly eastwards, and likewise that upon reaching Venice, the eastern half always veers suddenly northwards, and the two halves always rejoin and fuse together in Belluno, on their way — or rather, on
its
way — to Udine!
You may object that trains have no
inner
perspective on the matter — that “641” is just a third-person label rather than a first-person point of view. All I can say is, this is a very tempting viewpoint, but it is to be resisted. Trains
who
roll and trains
that
roll are the same thing, at least if they have sufficiently rich representational systems that allow them to wrap around and self-represent. Most trains today don’t (in fact none of them do), so we don’t usually give them the benefit of the “who” pronoun. But maybe someday they will, and then we will. However, the transition from one pronoun to the other won’t be sharp and sudden; it will be gradual, like the fading of the belief in Cartesian Egos as people grow in sophistication.
The Glow of the Soular Corona
It may strike you that this whole chapter has been predicated upon such weird science-fiction scenarios that it has no bearing at all upon how we think about the real world of real human beings, and their real lives and deaths. But I believe that that is mistaken.
I have a close friend whose aging father Jim has Alzheimer’s Disease. For some years my friend has been sadly watching his father lose contact, bit by bit, with one aspect after another of the reality that only a few years ago constituted the absolute bedrock, the completely reliable
terra firma,
of his inner life. He no longer knows his address, he has lost his former understanding of such mundane things as credit cards, and he isn’t quite sure who his children are, though they look vaguely familiar. And it is all getting dimmer, never brighter.
Perhaps Jim will forget his own name, where he grew up, what he likes to eat, and much more. He is heading into the same terrible, thick, allenveloping fog that former President Reagan lived in during the closing few low-huneker years of his life. And yet, something of Jim is surviving strongly — surviving in
other
brains, thanks to human love. His easy-going sense of humor, his boundless joy at driving the wide open spaces of the prairies, his ideals, his generosity, his simplicity, his hopes and dreams — and (for what it’s worth) his understanding of credit cards. All of these things survive at different levels in many people who, thanks to having interacted with him intimately over many years or decades, constitute his “soular corona” — his wife, his three children, and his many, many friends.
Even before Jim’s body physically dies, his soul will have become so foggy and dim that it might as well not exist at all — the soular eclipse will be in full force — and yet despite the eclipse, his soul will
still
exist, in partial, low-resolution copies, scattered about the globe. Jim’s first-person perspective will flicker in and out of existence in other brains, from time to time.
He
will exist, albeit in an extremely diluted fashion, now here, now there.
Where will Jim be?
Not very much anywhere, admittedly, but to some extent he will be in many places at once, and to different degrees. Though terribly reduced, he will be wherever his soular corona is.
It is very sad, but it is also beautiful. In any case, it is our only consolation.
CHAPTER 22
A Tango with Zombies and Dualism
Pedantic Semantics?
T
O ARGUE over whether the appropriate relative pronoun to apply to some hypothetical thinking machine one day in the future will be “who” or merely “which” would doubtless strike certain people as the quintessence of pedantic semantic quibbling, yet there are other people for whom the question would raise issues of life-or-death importance. Indeed, this is a quintessentially semantic issue, in that it involves deciding what verbal label to apply to something never seen before, but since category assignments go right to the core of thinking, they are determinant of our attitude toward each thing in the world, including such matters as life and death. For that reason, I feel that this pronoun issue, even if it is “merely semantics”, is of great importance to our sense of who or what we are.
The well-known Australian philosopher of mind David Chalmers, which not only is a cherished friend but also is my former doctoral student, has devoted many years to arguing for the provocative idea that there could be both “machines
that
think” and also “machines
who
think”. For me, the notion of both types of machine coexisting makes no sense, because, as I declared in Chapter 19, the word “thinking” stands for the dancing of symbols in a cranium or careenium (or some such arena), and this is also what is denoted by the word “consciousness”. Since being conscious merits the use of the pronoun “who” (and also, of course, the pronouns “I”, “me”, and so on), so does thinking — and that settles the question for me. In other words, “machine that thinks” is an incoherent phrase because of its relative pronoun, and if some day there really
are
machines that think, then by definition they will be machines
who
think.
Two Machines
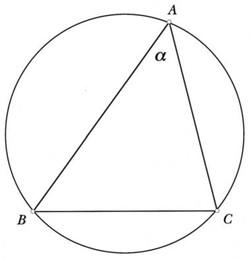
Dave Chalmers explores these issues in an unprecedented new fashion. He paints a picture of a world that has two machines identical down to the last nail, transistor, atom, and quark, and these two machines, sitting side by side on an old oaken table in Room 641 of the Center for Research into Consciousness and Cognetics at Pakistania University, are carrying out exactly the same task. For concreteness’ sake, let’s say both machines are struggling to prove, using informal geometrical insights rather than formal algebraic manipulations, the simple but surprising “chord–angle theorem” of Euclidean geometry, which states that if a point (
A
in the figure below) moves along an arc of a circle, then the angle (
α
) subtended by a fixed chord (
BC
) that the point is “looking at” as it moves along will be constant.
I chose this elementary but elegant theorem because it is one that Dave and I discussed together with great pleasure many years ago, and some of his comments on it gave me insights that literally changed my life. In fact, that fateful fork in the road way back when allows me to imagine Switch #6, the throwing of which would subtract from my brain all knowledge of this theorem and all the subsequent passion for geometry that was sparked by my thinking carefully about it…
As I was saying, these two exactly identical machines are launched on this task in the exact same terasecond by an atomic clock, and they proceed in exact lockstep synchrony towards its solution, simulating, let us say, the exact processes that took place in Dave Chalmers’ own brain when he first found an insight-yielding visual proof. The details of the program running in both machines are of no import to us here; what does matter is that Machine Q (it stands for “qualia”) is actually
feeling
something, whereas Machine Z (it rhymes with “dead”) is feeling nothing. This is where Dave’s ideas grow incomprehensible to me.
Now I have to admit that in order to make it a bit easier to envision, I have slightly altered the story that Dave tells. I placed these two machines side by side on the old oaken table in Room 641 of CRCC, while Dave never does that. In fact, he would protest, saying something such as, “It’s bloody incoherent to postulate two identical machines running identical processes on the very same oaken table with one of them feeling something and the other one not. That violates the laws of the universe!”
I fully accept this objection and plead guilty to having distorted Dave’s tale. To atone for my sin and to turn my story back into his, I first remove one of the machines from the old oaken table in Room 641. Let’s call the machine who remains, no matter what we’d called it before, “Machine Q”. Now (following Dave), we take a rather unexpected step: we imagine a different but isomorphic (
i.e.,
“separate but indistinguishable”) universe. We’ll call the first one “Universe Q” and the new one “Universe Z”. Both universes have exactly the same laws of physics, and in each universe the laws of physics are all one needs to know in order to predict what will happen, given any initial configuration of particles.
