Gödel, Escher, Bach: An Eternal Golden Braid (131 page)
Read Gödel, Escher, Bach: An Eternal Golden Braid Online
Authors: Douglas R. Hofstadter
Tags: #Computers, #Art, #Classical, #Symmetry, #Bach; Johann Sebastian, #Individual Artists, #Science, #Science & Technology, #Philosophy, #General, #Metamathematics, #Intelligence (AI) & Semantics, #G'odel; Kurt, #Music, #Logic, #Biography & Autobiography, #Mathematics, #Genres & Styles, #Artificial Intelligence, #Escher; M. C

So now we make a modification in our robot: we allow its symbols--including its selfsymbol-to affect the decision that is taken. Now here is an example of a program running fully under physical law, which seems to get much more deeply at the essence of choice than the previous examples did. When the robot's own chunked concept of itself enters the scene, we begin to identify with the robot, for it sounds like the kind of thing we do. It is no longer like the calculation of the square root of 2, where no symbols seem to be monitoring the decisions taken. To be sure, if we were to look at the robot's program on a very local level, it would look quite like the square-root program. Step after step is executed, and in the end "left" or "right" is the output. But on a high level we can see the fact that symbols are being used to model the situation and to affect the decision. That radically affects our way of thinking about the program. At this stage,
meaning
has entered this picture-the same kind of meaning as we manipulate with our own minds.
A Gödel Vortex Where All Levels Cross
Now if some outside agent suggests `L' as the next choice to the robot, the suggestion will be picked up and channeled into the swirling mass of interacting symbols. There, it will be sucked inexorably into interaction with the self-symbol, like a rowboat being pulled into a whirlpool. That is the vortex of the system, where all levels cross. Here, thèL' encounters a Tangled Hierarchy of symbols and is passed up and down the levels. The self-symbol is incapable of monitoring all its internal processes, and so when the actual decision emerges-'L' or 'R' or something outside the system-the system will not be able to say where it came from. Unlike a standard chess program, which does not monitor itself and consequently has no ideas about where its moves come from, this program does monitor itself and does have ideas about its ideas-but it cannot monitor its own processes in complete detail, and therefore has a sort of intuitive sense of its workings, without full understanding. From this balance between self-knowledge and self-ignorance comes the feeling of free will.
Think, for instance, of a writer who is trying to convey certain ideas which to him are contained in mental images. He isn't quite sure how those images fit together in his mind, and he experiments around, expressing things first one way and then another, and finally settles on some version. But does he know where it all came from? Only in a vague sense. Much of the source, like an iceberg, is deep underwater, unseen-and he knows that. Or think of a music composition program, something we discussed earlier, asking when we would feel comfortable in calling it the composer rather than the tool of a human composer. Probably we would feel comfortable when self-knowledge in terms of symbols exists inside the program, and when the program has this delicate balance between self-knowledge and self-ignorance. It is irrelevant whether the system is running deterministically; what makes us call it a "choice maker" is
whether we can identify with
a high-level description of the process which takes place when the
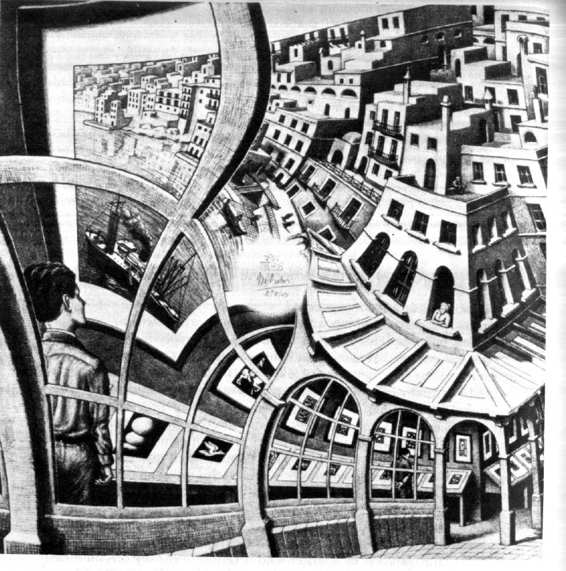
FIGURE 142.
Print Gallery, by M. C. Escher (lithograph, 1956)
.
program runs
. On a low (machine language) level, the program looks like any other program; on a high (chunked) level, qualities such as "will", "intuition", "creativity", and
"consciousness" can emerge.
The important idea is that this "vortex" of self is responsible for the tangledness, for the Gödelian-ness, of the mental processes. People have said to me on occasion, "This stuff with self-reference and so on is very amusing and enjoyable, but do you really think there is anything serious to it?" I certainly do. I think it will eventually turn out to be at the core of AI, and the focus of all attempts to understand how human minds work. And that is why Godel is so deeply woven into the fabric of my book.
An Escher Vortex Where All Levels Cross
A strikingly beautiful, and yet at the same time disturbingly grotesque, illustration of the cyclonic "eye" of a Tangled Hierarchy is given to us by Escher in his
Print Gallery
(Fig.
142). What we see is a picture gallery where a young man is standing, looking at a picture of a ship in the harbor of a small town, perhaps a Maltese town, to guess from the architecture, with its little turrets, occasional cupolas, and flat stone roofs, upon one of which sits a boy, relaxing in the heat, while two floors below him a woman-perhaps his mother-gazes out of the window from her apartment which sits directly above a picture gallery where a young man is standing, looking at a picture of a ship in the harbor of a small town, perhaps a Maltese town--What!? We are back on the same level as we began, though all logic dictates that we cannot be. Let us draw a diagram of what we see (Fig.
143).
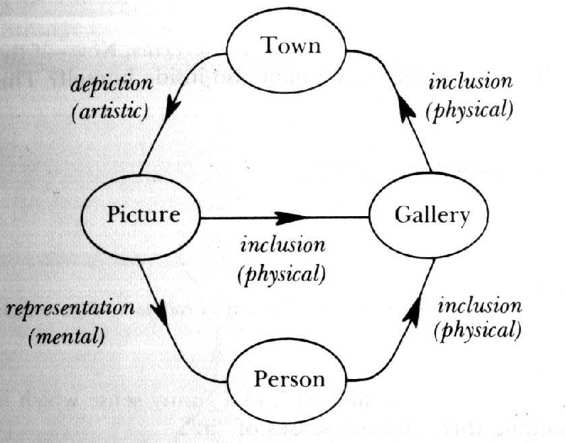
FIGURE 143.
Abstract diagram of M. C. Escher's Print Gallery
.
What this diagram shows is three kinds of "in-ness". The gallery is
physically in
the town ("inclusion"); the town is
artistically
in the picture ("depiction"); the picture is
mentally
in the person ("representation"). Now while this diagram may seem satisfying, in fact it is arbitrary, for the number of levels shown is quite arbitrary. Look below at another way of representing the top half alone (Fig. 144).
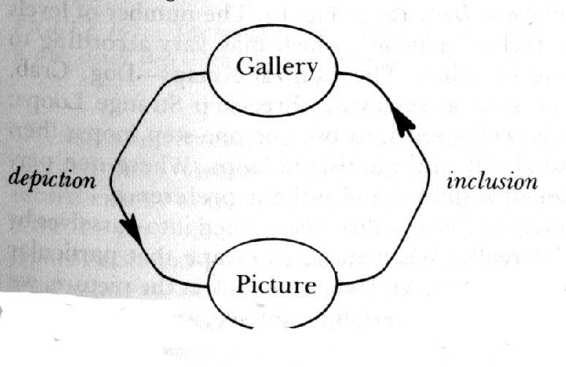
FIGURE 144.
A collapsed version of the previous figure.
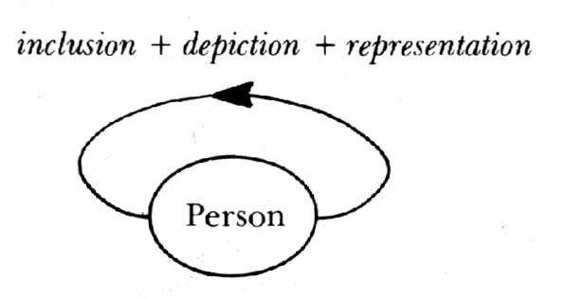
We have eliminated the "town" level; conceptually it was useful, but can just as well be done without. Figure 144 looks just like the diagram for Drawing Hands: a Strange Loop of two steps. The division markers are arbitrary, even if they seem natural to our minds.
This can be further accentuated by showing even more "collapsed" schematic diagrams of
Print Gallery
, such as that in Figure 145.
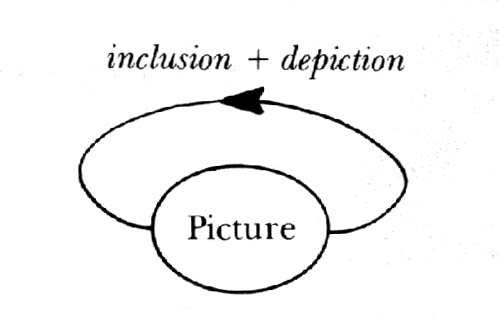
FIGURE 145.
Further collapse of Figure 143
.
This exhibits the paradox of the picture in the starkest terms. Now-if the picture is "inside itself", then is the young man also inside himself-, This question is answered in Figure 146.
FIGURE 146.
Another way of collapsing Figure 143.
Thus, we see the young man "inside himself", in a funny sense which is made up of compounding three distinct senses of "in".
This diagram reminds us of the Epimenides paradox with its one-step selfreference, while the two-step diagram resembles the sentence pair each of which refers to the other. We cannot make the loop any tighter, but we can open it wider, by choosing to insert any number of intermediate levels, such as "picture frame", "arcade", and
"building". If we do so, we will have many-step Strange Loops, whose diagrams are isomorphic to those of
Waterfall
(Fig. 5) or
Ascending and Descending
(Fig. 6). The number of levels is determined by what we feel is "natural", which may vary according to context, purpose, or frame of mind. The Central Xmaps-Dog, Crab, Sloth, and Pipe-can all be seen as involving three-step Strange Loops; alternatively, they can all be collapsed into two- or one-step loops;. then again, they can be expanded out into multistage loops.
Where one perceives the levels is a matter of intuition and esthetic preference.
Now are we, the observers of Print Gallery, also sucked into ourselves by virtue of looking at it? Not really. We manage to escape that particular vortex by being outside of the system. And when we look at the picture, we see things which the young man can certainly not see, such as Escher's
Signature, "MCE", in the central "blemish". Though the blemish seems like a defect, perhaps the defect lies in our expectations, for in fact Escher could not have completed that portion of the picture without being inconsistent with the rules by which he was drawing the picture. That center of the whorl is-and must be-incomplete. Escher could have made it arbitrarily small, but he could not have gotten rid of it. Thus we, on the outside, can know that
Print Gallery
is essentially incomplete-a fact which the young man, on the inside, can never know. Escher has thus given a pictorial parable for Gödel’s Incompleteness Theorem. And that is why the strands of Gödel and Escher are so deeply interwoven in my book.
